solve for x tan inverse(2x/1x^2) cot inverse (1x^2/2x)= pi/3, 1 Next Integration Formula Sheet Chapter 7 Class 12 Formulas→ Chapter 7 Class 12 Integrals (Term 2) Serial order wise;For each of the three trigonometric substitutions above we will verify that we can ignore the absolute value in each case when encountering a radical 🔗 For x = asinθ, x = a sin θ, the expression √a2 −x2 a 2 − x 2 becomes √a2−x2 = √a2−a2sin2θ= √a2(1−sin2θ)= a√cos2θ= acosθ = acosθ a 2 − x 2 = a 2 − a 2
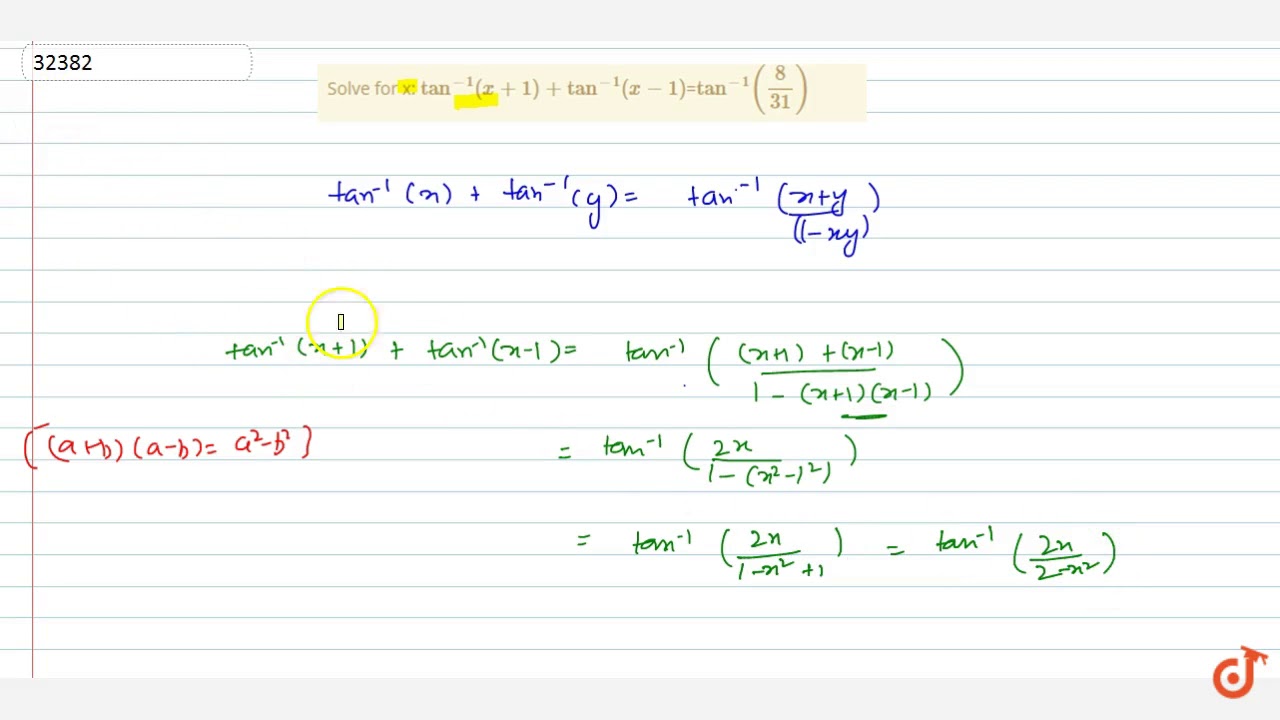
Solve For X Tan 1 X 1 Tan 1 X 1 Tan 1 8 31 Youtube
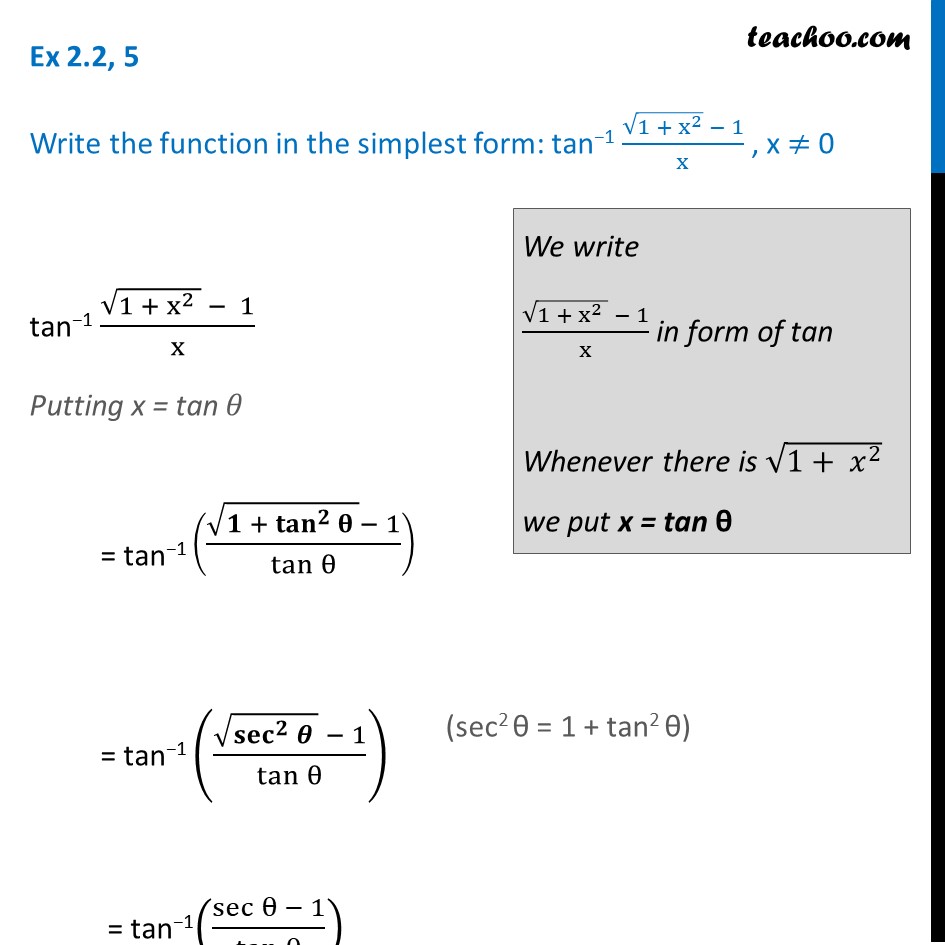
Tan inverse 2x/1-x^2 formula
Tan inverse 2x/1-x^2 formula-Tan 1 (2x/ (1x 2 )=2tan 1 x cot 1 ( (1x 2 )/2x 2 )=tan 1 (2x 2 / (1x 2 )) use the formula tan 1 a tan 1 b =tan 1 ( (ab)/ (1ab)) Approved ankit dewangan 32 Points 9 years ago now i have only days to my board exams suggest some tricksTan^1 x Not sure if you mean arctan x or (tan x)^1 which is cot x (i) y = arctan x Then, tan y = x Differentiating implicitly wrt x sec^2 y 1 dy/dx = 1 sec^2 y dy/dx = 1 dy/dx = 1/sec^2 y And, tan y = x , so, tan^2 y = x^2 sec^2 y = 1
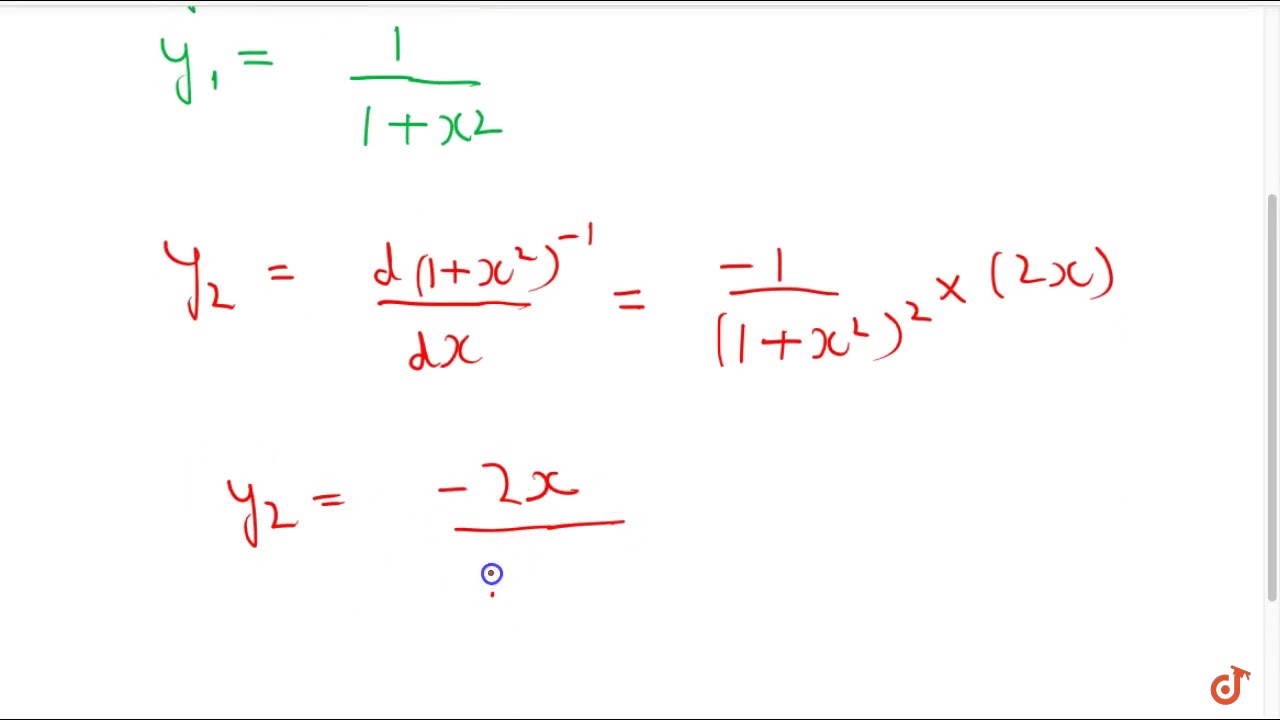



If Y Tan 1 X Show That 1 X 2 D 2y Dx 2 2x Dy Dx 0 Youtube
Solve the following equations for x 2 tan^1(sin x) = tan^1 (2 sec x), x ≠ π/2 asked Apr 2 in Trigonometry by Takshii ( 346k points) inverse trigonometric functionsMiscellaneous Misc 1 Important Misc 2 Important Misc 3 Important Misc 4 Misc 5 Important Misc 6Answer with step by step detailed solutions to question from HashLearn's Math CPPs (JEE), Integral Calculus "integral 2x1/( x1 )(x^21 ) dx" plus more questions from Mathematics Questions of this type are frequently asked in competitive entrance
Function of x, not of y We must now plug in the original formula for y, which was y = tan−1 x, to get y = cos2(arctan(x)) This is a correct answer but it can be simplified tremendously We'll use some geometry to simplify it 1 x (1x2)1/2 y Figure 3 Triangle with angles and lengths corresponding to those in the exam pleWhole number n if $sin\ θ=sin\ a,$ then $θ=nπ(1)^n\ a$ if $cosec\ θ=cosec\ a,$ then $θ=nπ(1)^n\ a$ if $cos\ θ=cos\ a,$ then $θ=2nπ±a$ if $sec\ θ=secLet P = a i j be a 3 × 3 matrix and let Q = b i j where b i j = 2 i j a i j for 1 ≤ i, j ≤ If the determinant of P is 2, then the determinant of the matrix Q is 5 If the sum of n terms of an AP is given by S n = n 2 n, then the common difference of the AP is
Then by using the formula used for solving functions in parametric form ie Lastly substituting the values of du/dx and dv/dx and simplify to obtain the result Calculation Let u = tan1 x and v = cot1 x Differentiating with respect to x, we get \(\rm \frac{du}{dx} = \frac{d(\tan^{1Integral of tan^2 (x) \square! Prove that tan^1x tan^1(2x/(1 x^2)) = tan^1((3x x^3)/(1 3x^2)) asked in Trigonometry by KumkumBharti ( 539k points) inverse trigonometric functions



Www Southhadleyschools Org Cms Lib Ma Centricity Domain 11 5 7 Pdf
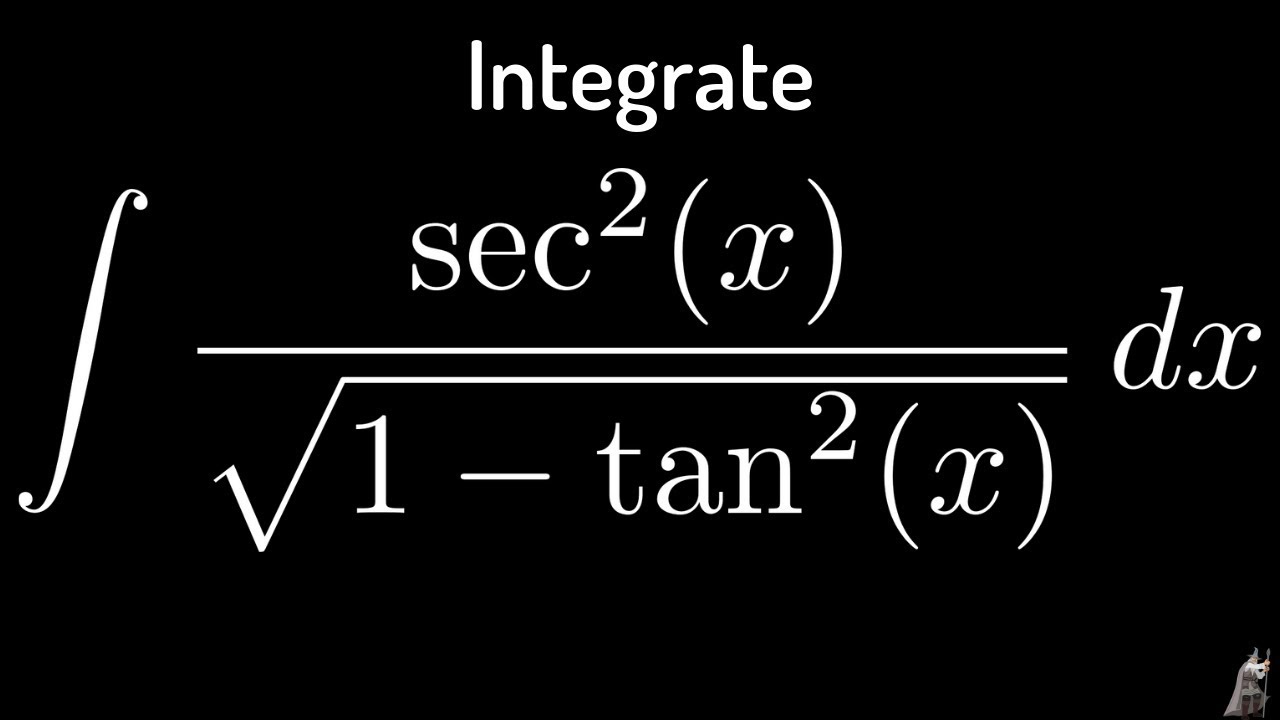



Integral Of Sec 2 X Sqrt 1 Tan 2 X Using The Arcsine Function Youtube
Solve the Following Equation For X `Tan^1 X/2Tan^1 X/3=Pi/4, 0Cos Inverse 1 X 2 1 X 2 Formula Solve For X Cos 1 X 2 1 X 2 1 1 2tan 1 2x 1 X 2 2pi 3 Youtube For more information and source, see on this link https Solve For X Tan 1 2x 1 X 2 Cot 1 1 X 2 2x P 3 1 X 1 Sarthaks Econnect Largest Online Education CommunityTan1 x = Cot1 1/x Formula 3 The sum of the complementary inverse trigonometric functions results in a right angle For the same values of x, the complementary functions have complementary angles as answers, and the sum of it is equal to a right angle Sin1 x Cos1 x = π/2 Tan1 x Cot1 x = π/2 Sec1 x Cosec1 x = π/2 Formula 4
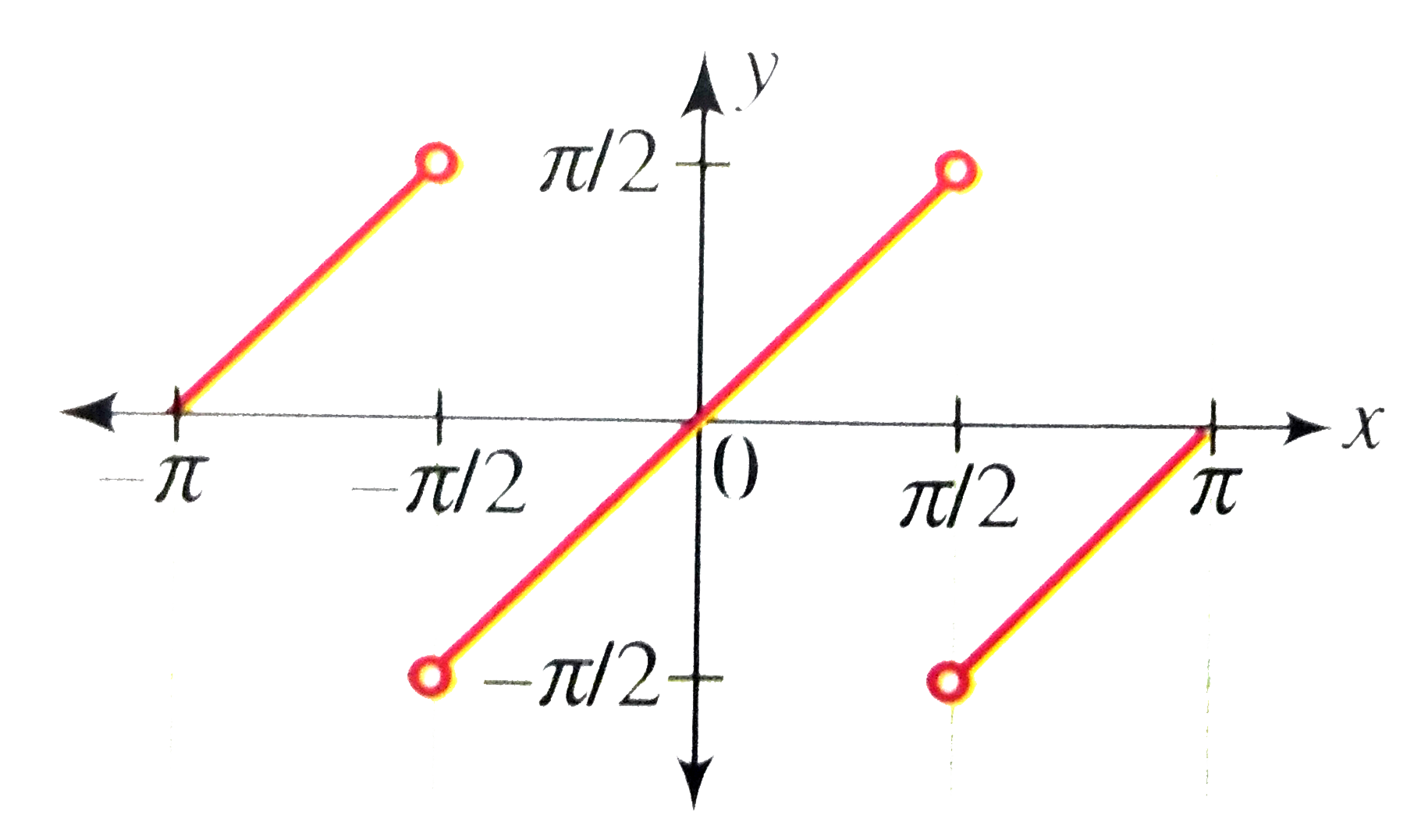



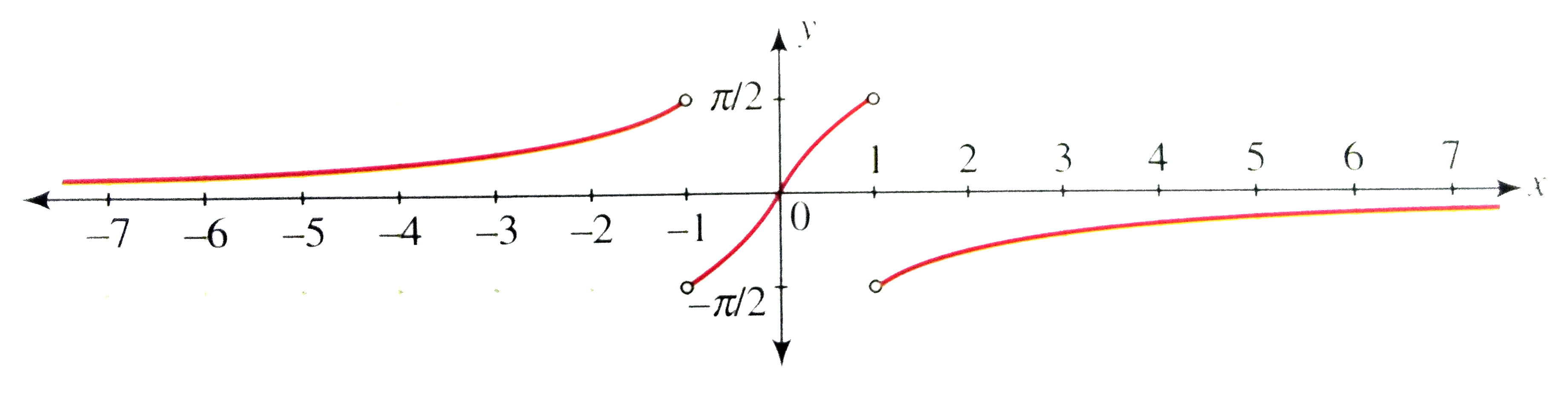
Draw The Graph Of Y Tan 1 2x 1 X 2




Tan Inverse 2x 1 X Square Cot Inverse 1 X Square 2x 2pi 3 Brainly In
Differentiate wrtx du/dx = 2/ (1 x 2) Let v = sin 1 2x/ (1x 2) Put x = tan θ So v = sin 1 2 tan θ/ (1 tan 2 θ) = sin 1 sin 2θ = 2θ = 2 tan 1 x dv/dx = 2/ (1 x 2)Algebra Factoring Formula Product Formula Power Formula Logarithm Formula Equation and Solutions Inequalities Compound Interest Formula Root Formula TrigonometryGet stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!
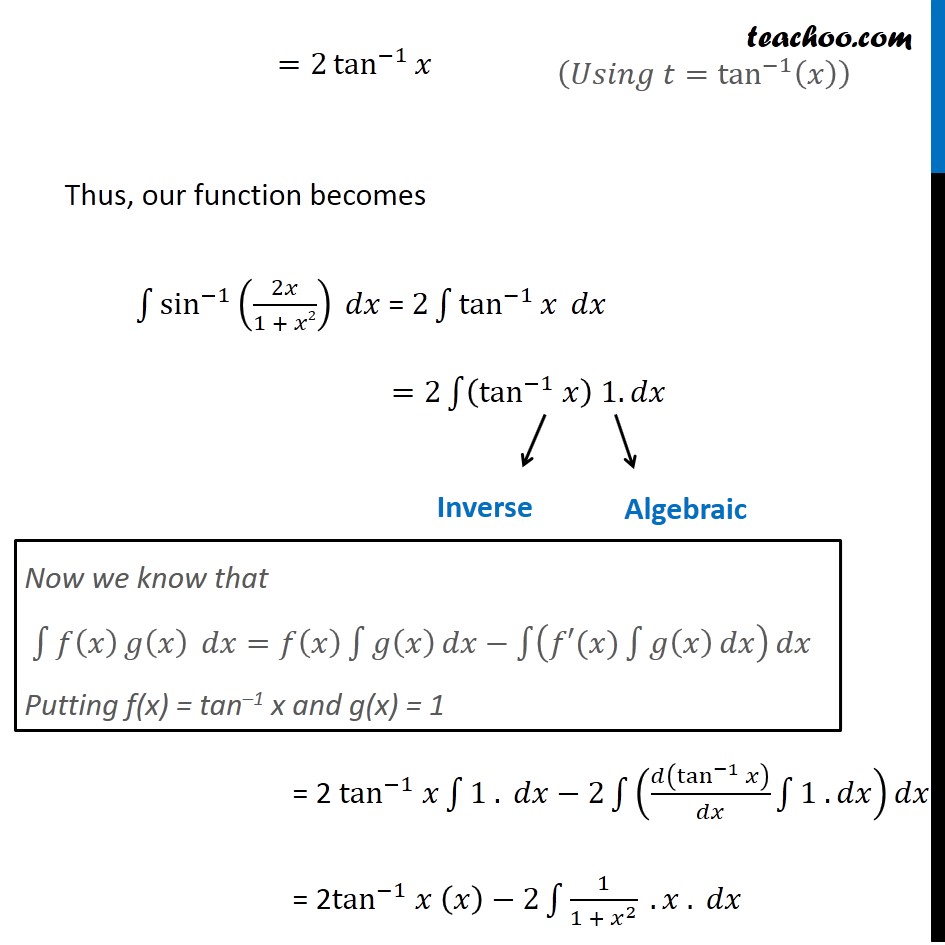



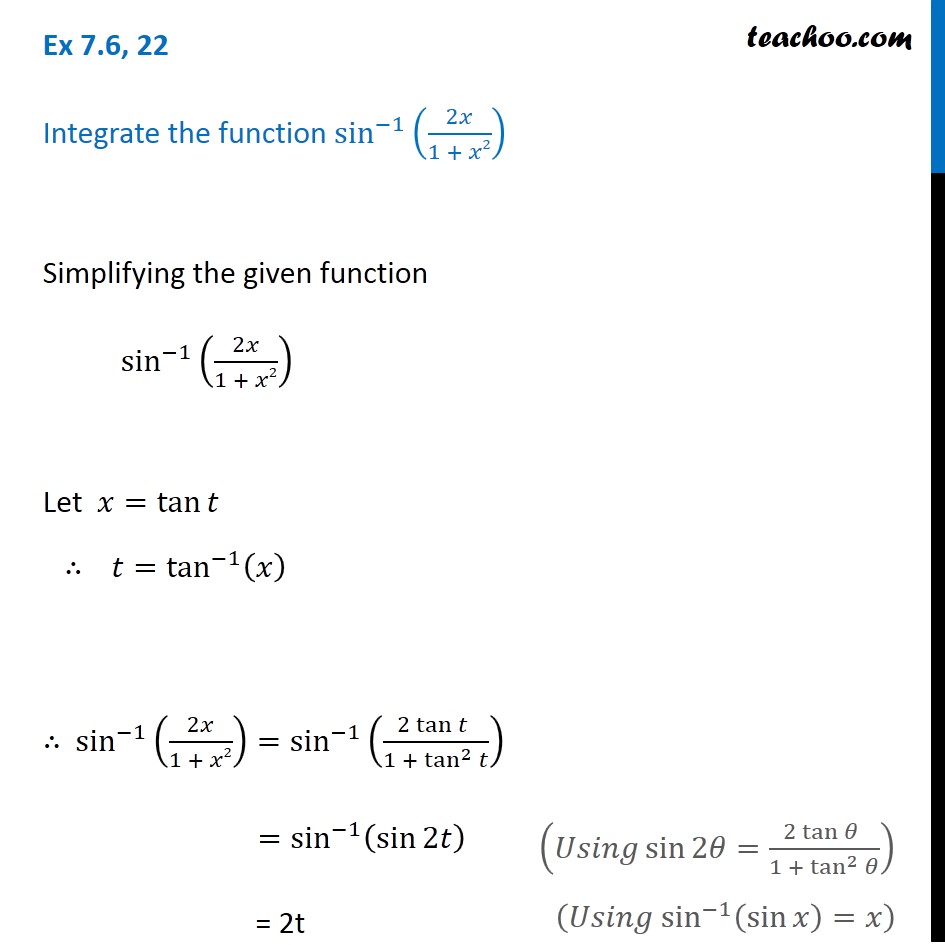
Ex 7 6 22 Integrate Sin 1 2x 1 X 2 Teachoo Ex 7 6
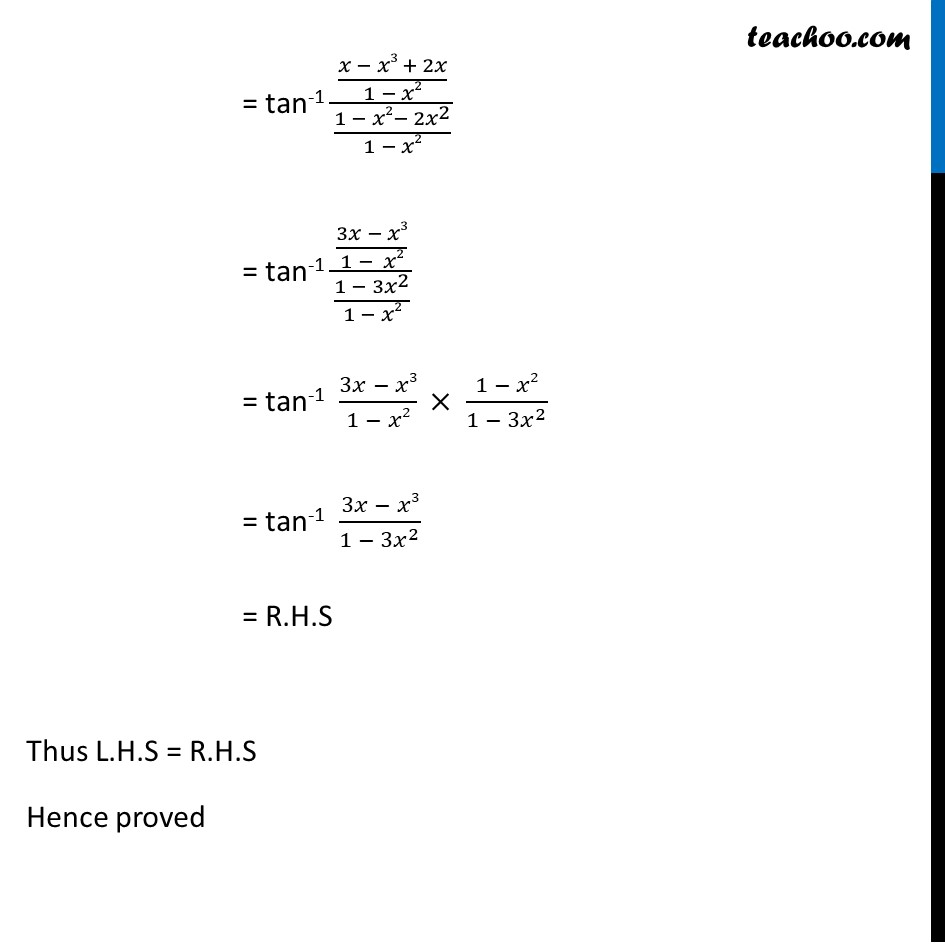



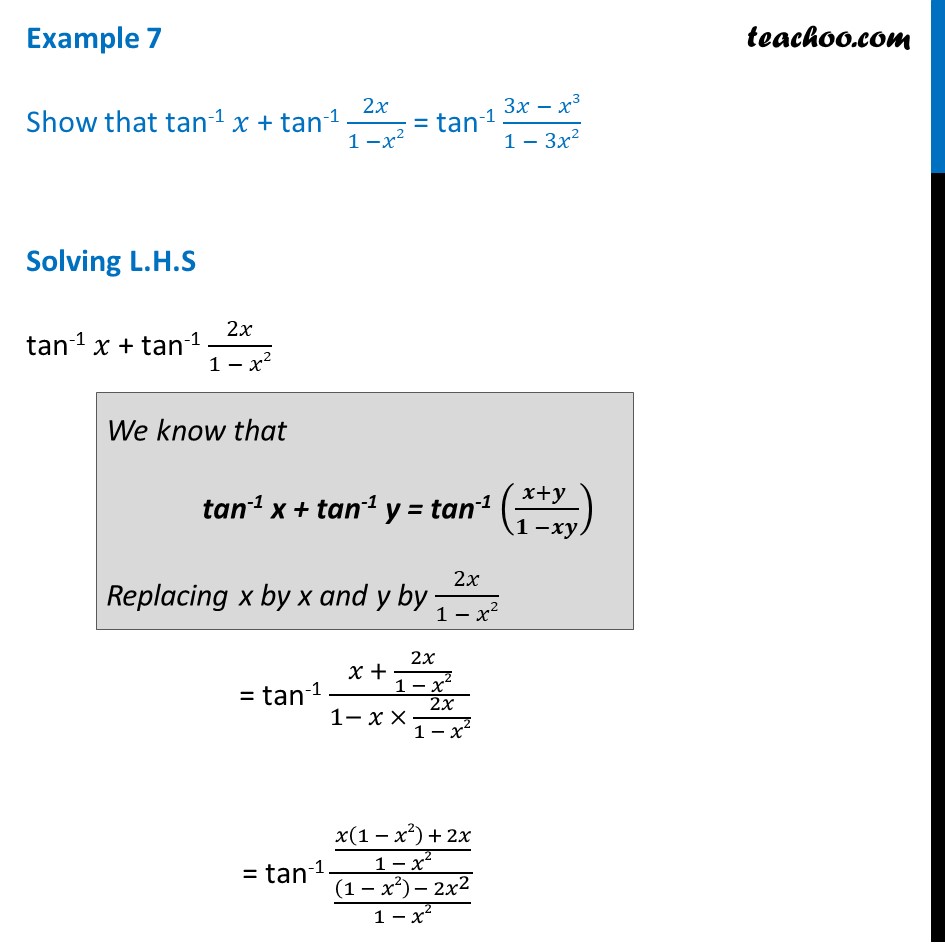
Example 7 Show That Tan 1 X Tan 1 2x 1 X2 Inverse
This means ∫π 0 sin(x)dx= (−cos(π))−(−cos(0)) =2 ∫ 0 π sin ( x) d x = ( − c o s ( π)) − ( − c o s ( 0)) = 2 Sometimes an approximation to a definite integral is desired A common way to do so is to place thin rectangles under the curve and add the signed areas together WolframAlpha can solve a broad range of integralsINVERSE TRIGONOMETRIC FUNCTIONS 23 Therefore, tan(cos–1x) = 1–cos θ 21– tanθ = cosθ x x = Hence 2 –1 8 1– 8 17 15 tan cos = 17 8 8 17 = Example 11 Find the value of –1 –5 #tan^1(xsqrt(1x^2))#,Express the value of the above in the simplest form?




How Do You Find The Equations Of The Tangent Lines To The Curve Y X 1 X 1 That Are Parallel To The Line X 2y 2 Socratic




Derive The Following Formulas For The Inverse Chegg Com
The integration of tangent inverse is of the form I = ∫ tan – 1 x d x To solve this integration, it must have at least two functions, however it has only one function tan – 1 x So, consider the second function as 1 Now the integration becomes I = ∫ tan – 1 x ⋅ 1 d x – – – ( i) The first function is tan – 1 x and theBefore reading this, make sure you are familiar with inverse trigonometric functions The following inverse trigonometric identities give an angle in different ratios Before the more complicated identities come some seemingly obvious ones Be observant of the conditions the identities call for sin − 1 ( − x) = − sin − 1 x, ∣Detailed explanation with examples on propertiesofinversetrigonometricfunctions helps you to understand easily , designed as per NCERT QnA , Notes & Videos




Notes On A Level P3 Integration Notes



1
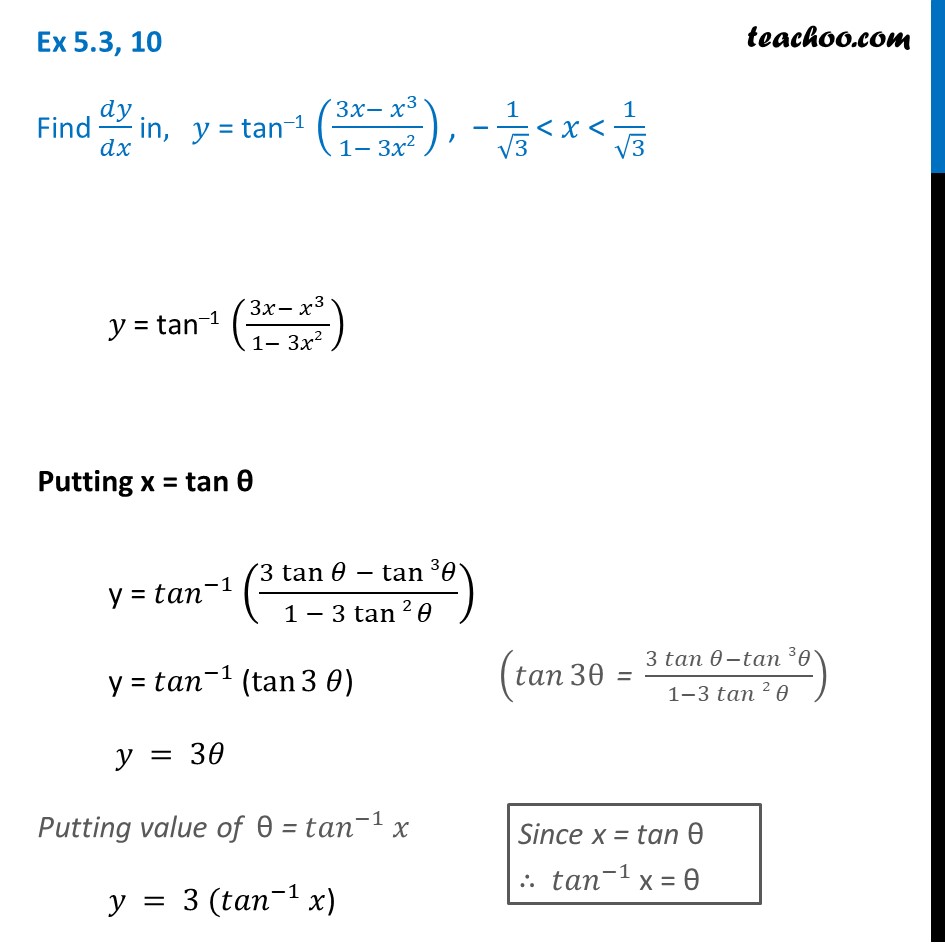
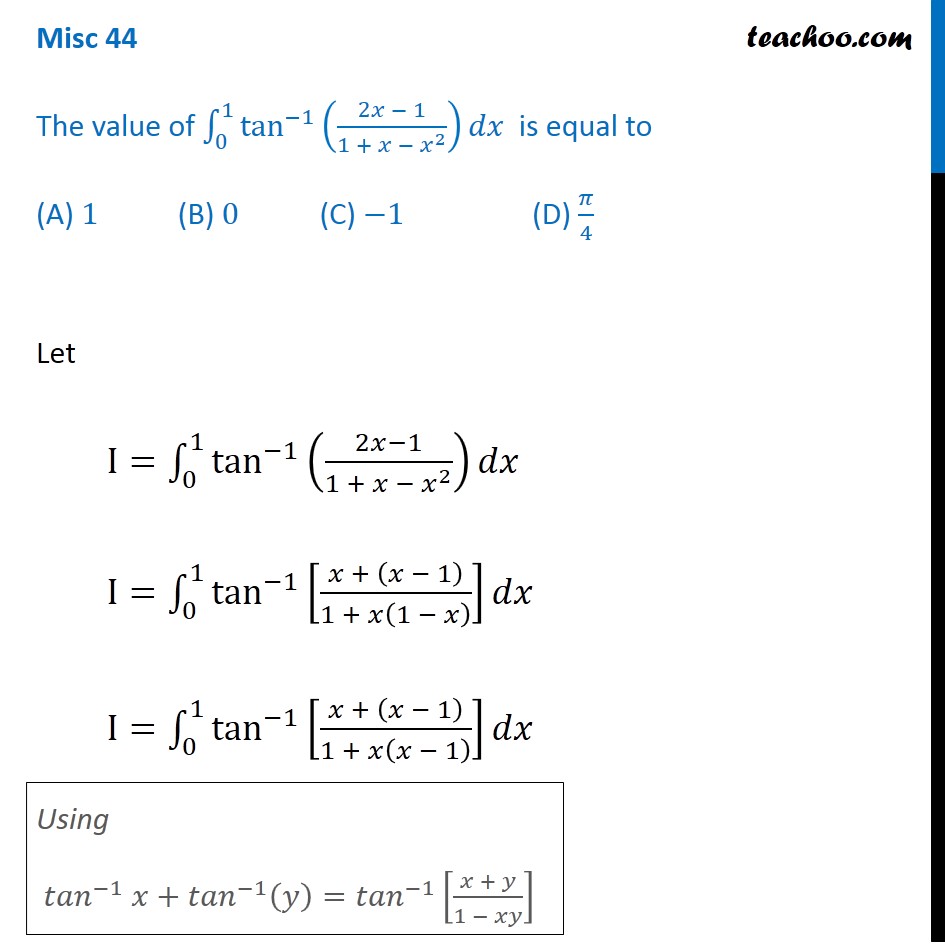
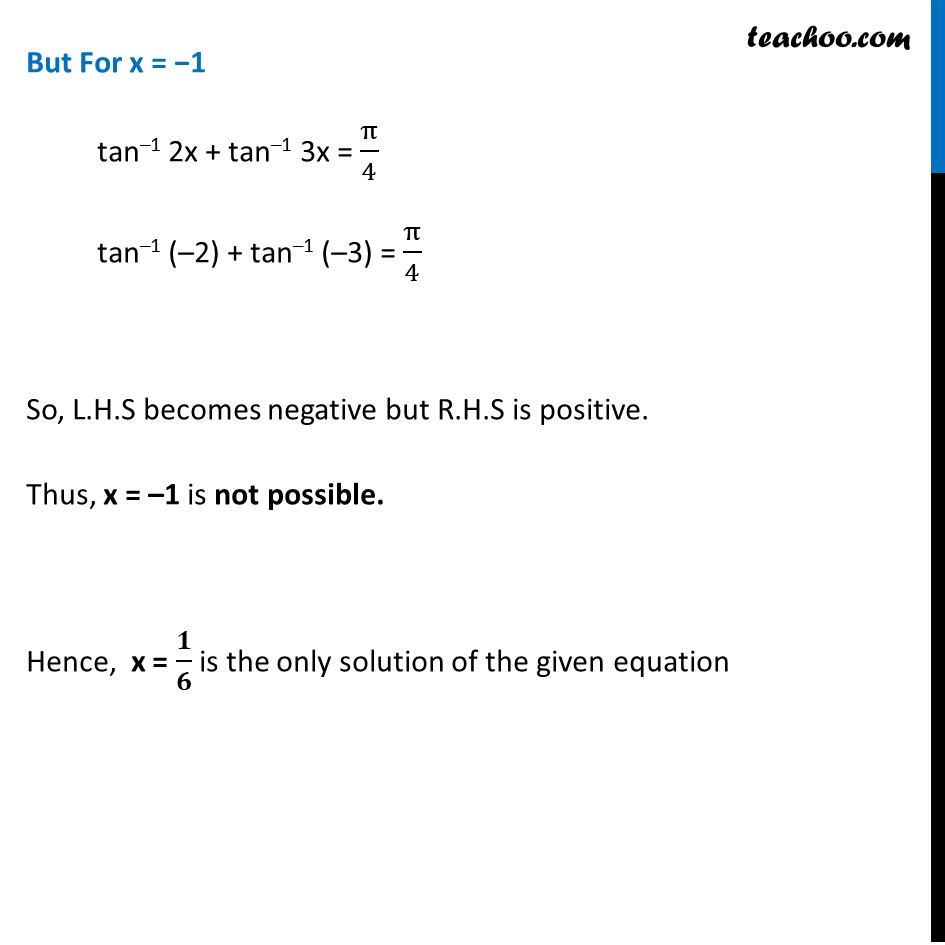
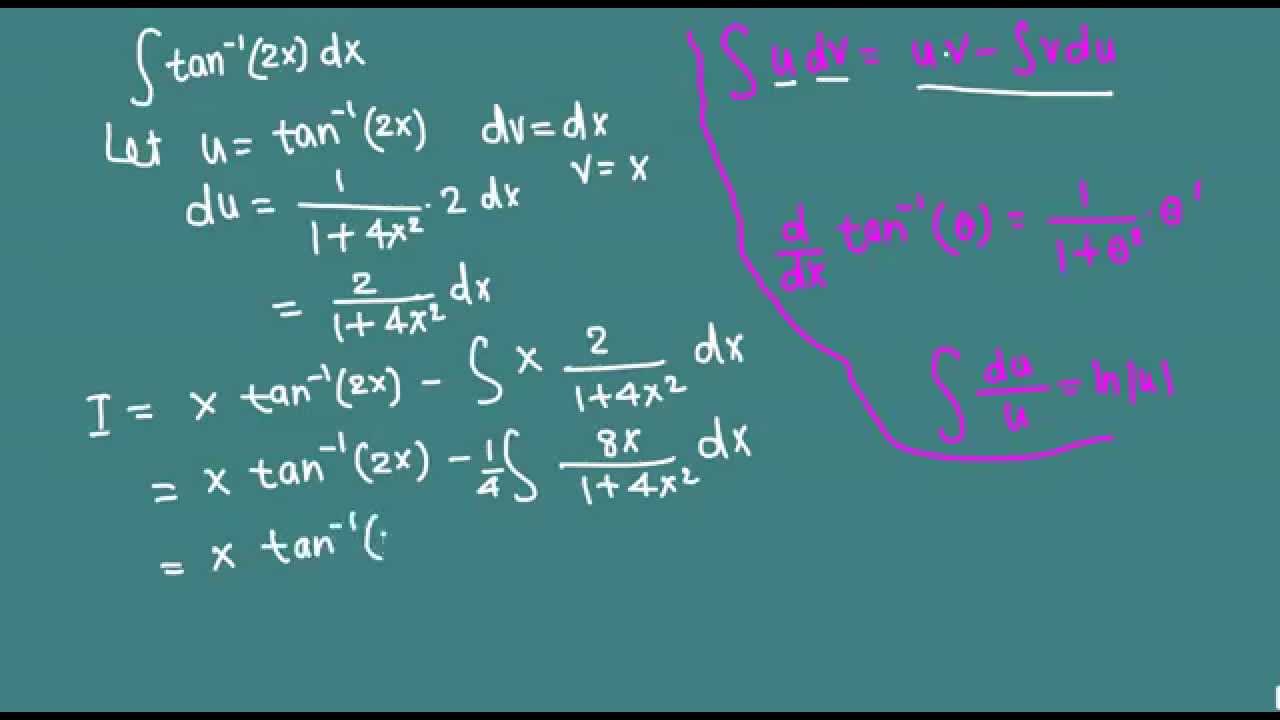
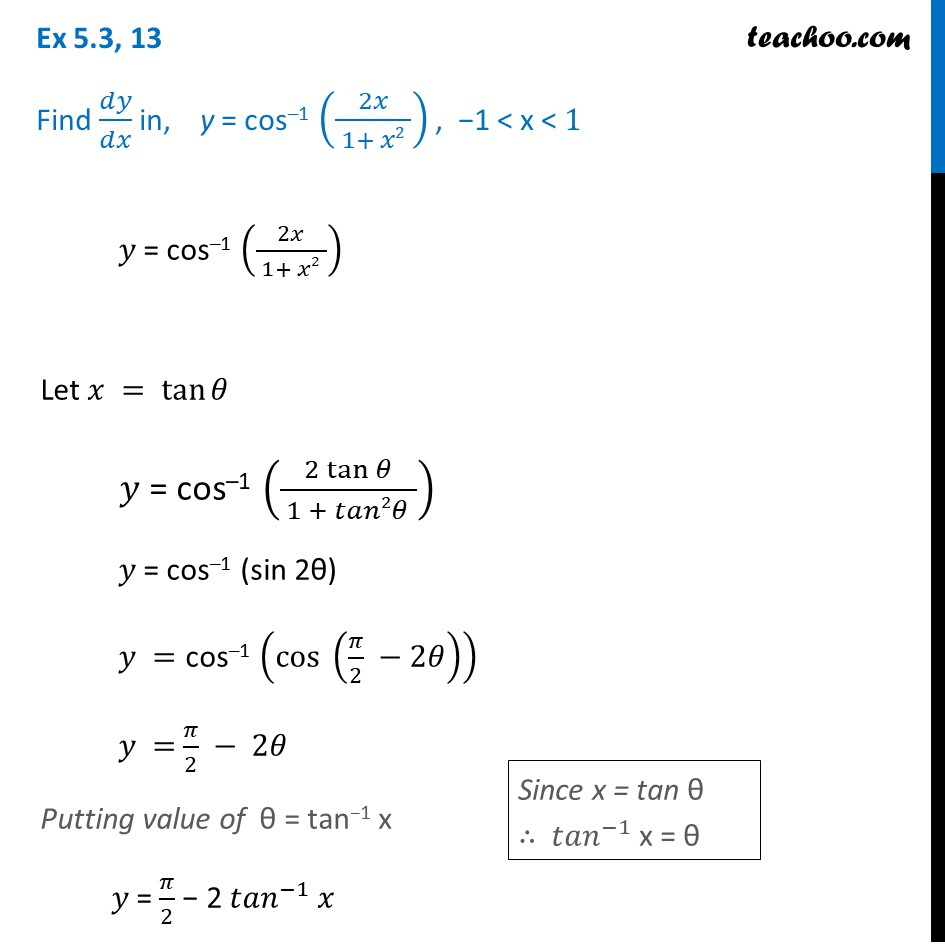
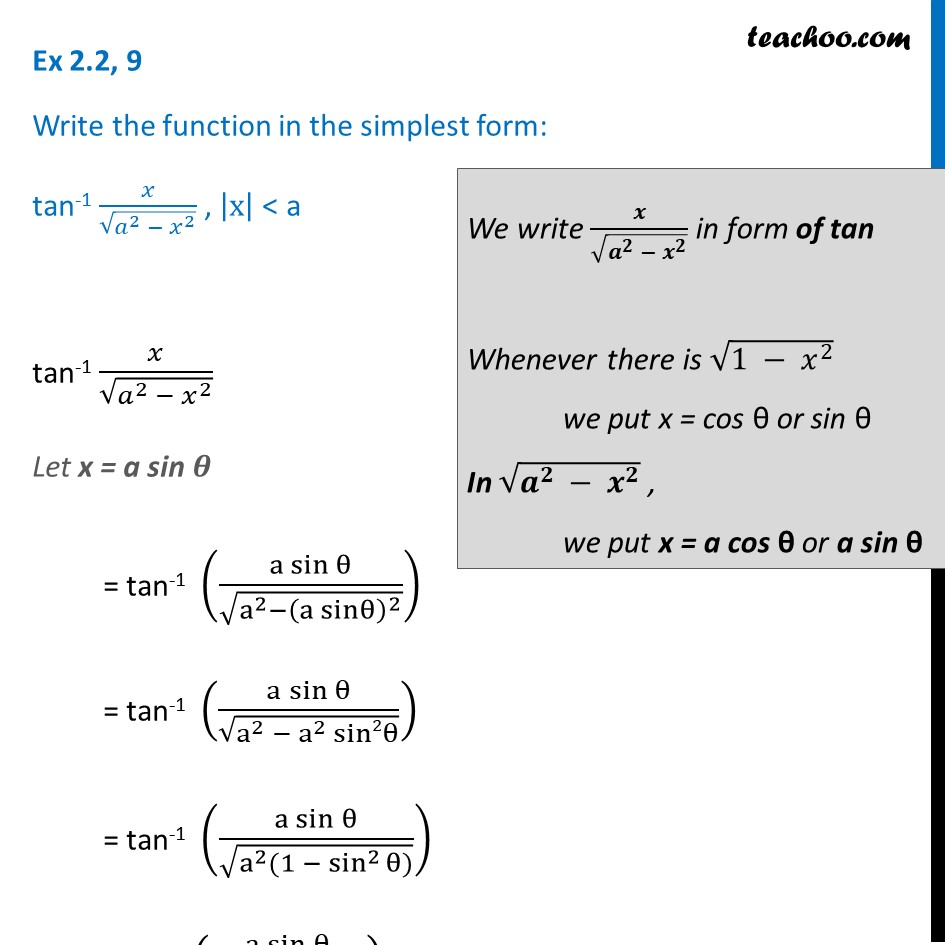
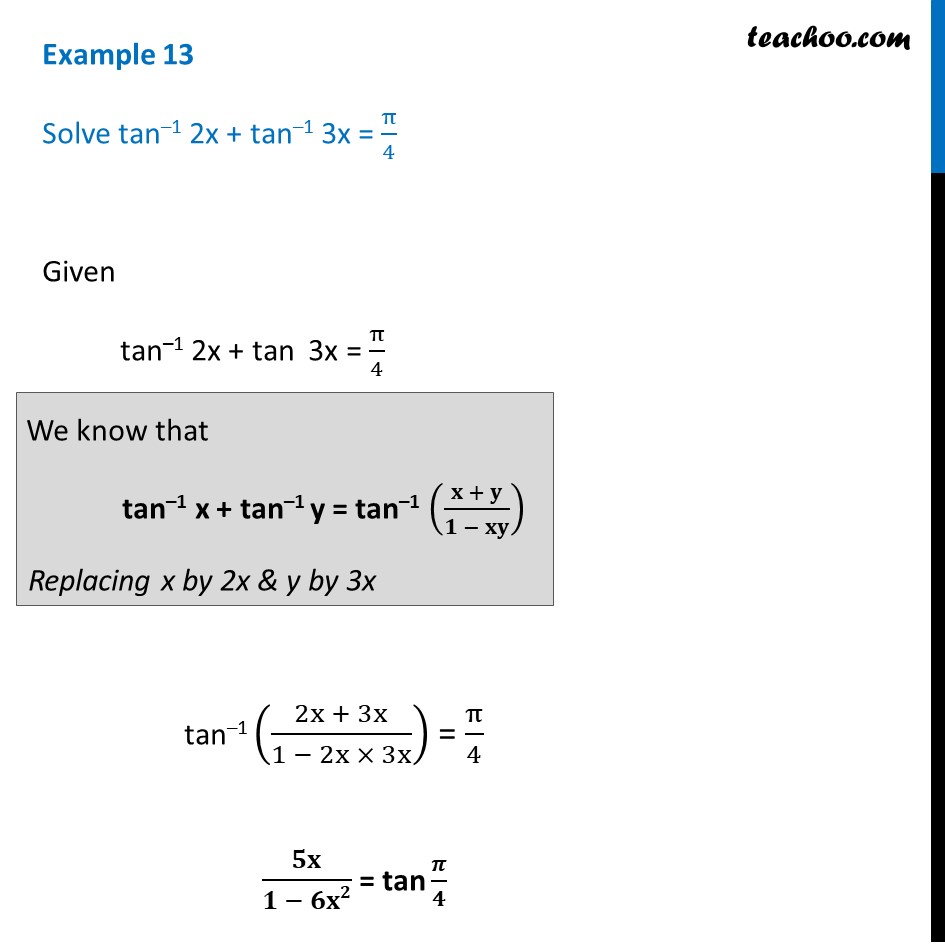
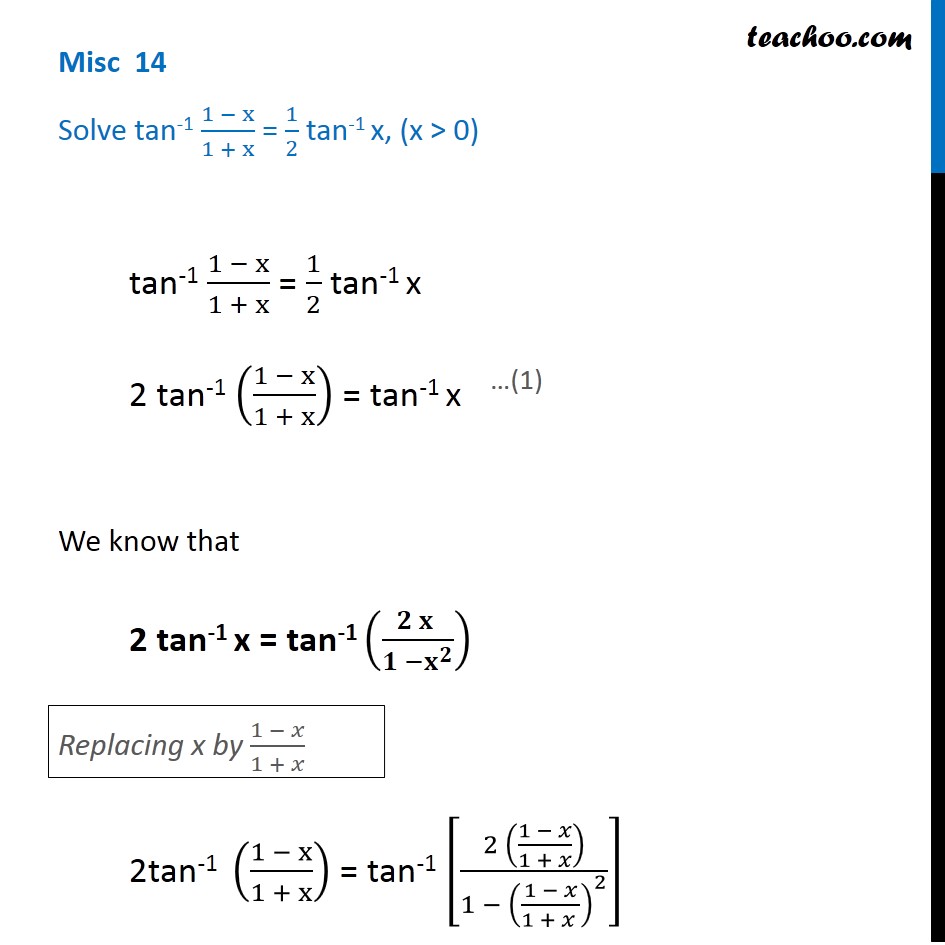
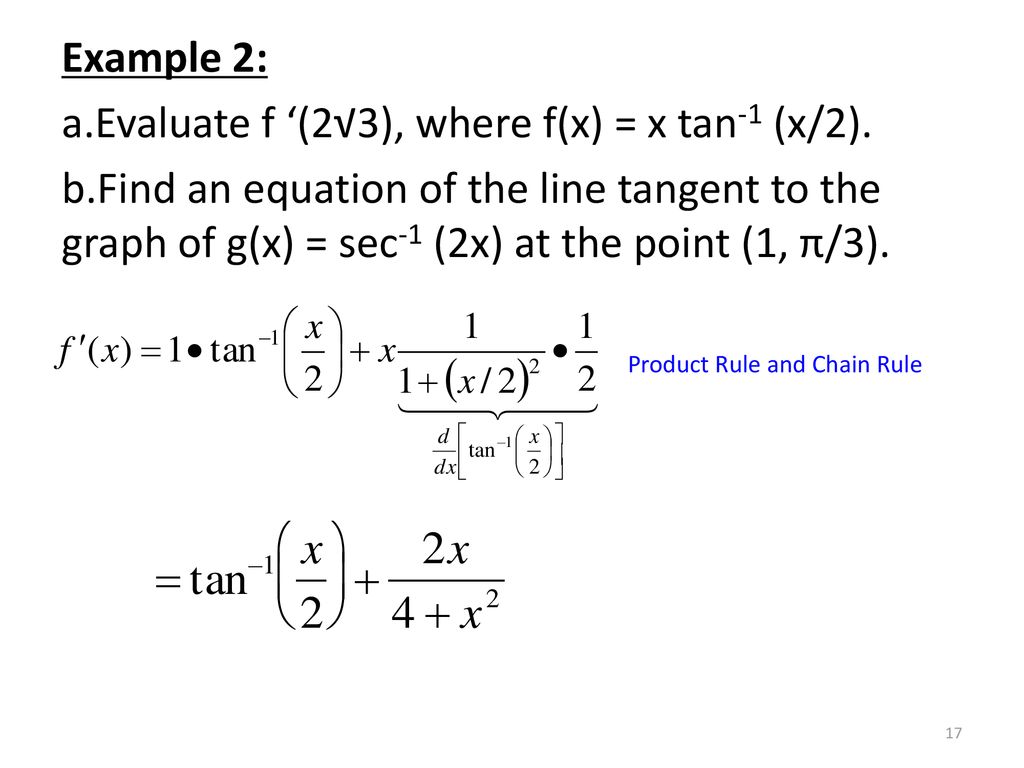
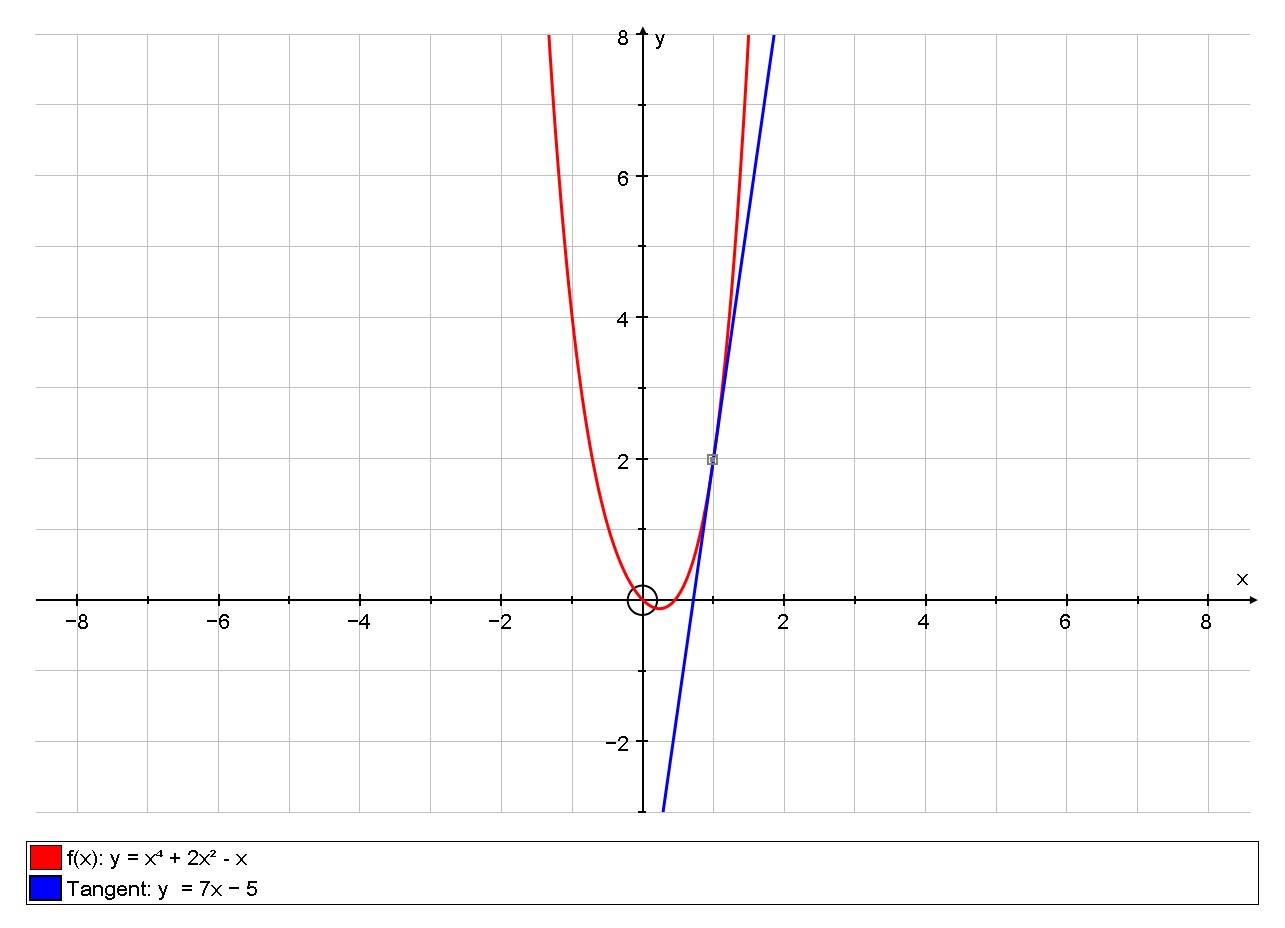
Solve for x tan^1 (x 1) tan^1 x tan^1 (x 1) = tan^1 3xExample 1 In the integral we may write = , = , = , so that the integral becomes = = ( ) = = = = , provided For a definite integral, the bounds change once the substitution is performed and are determined using the equation = , with values in the range Derivatives Of Inverse Functions Video Khan Academy Solve For X Tan 1 X 1 Tan 1 X 1 Tan 1 8 31 Youtube The inverse trigonometric functions are also called arcus functions or anti trigonometric functions These are the inverse functions of the trigonometric functions with suitably restricted domainsSpecifically, they are the inverse functions of the sine, cosine, tangent, cotangent, secant, and cosecant functions, and are used to obtain an angle from any of the angle's trigonometricLimit Calculator Step 1 Enter the limit you want to find into the editor or submit the example problem The Limit Calculator supports find a limit as x approaches any number including infinity The calculator will use the best method available so try out a lot of different types of problems You can also get a better visual and understanding Solution Let x = sec θ, then x 2 − 1 = s e c 2 θ − 1 = tan θ Therefore, cot–1= 1 x 2 – 1 = cot–1 (cot θ) = θ = sec–1 x, which is the simplest form Inverse trigonometric formula here deals with all the essential trigonometric inverse function which will make it easy for you to learn anywhere and anytime ITF formula for class 3 Differentiate Sin 1 2x 1 X 2 W R T Tan 1 X 1 Lt X Lt 1 The other answer so far (from Eshaan Joshe) should put you on your way, but be careful that that answer meant 1/(1x^2) If you continue taking the derivatives, you can look for a pattern (using arctan to mean tan^1) first derivative of (arctan Solve for `x` `tan^(1)(2x)/(1x^(2))cot^(1)(1x^(2))/(2x))=pi/3, 1 lt x lt 1`Notation Several notations for the inverse trigonometric functions exist The most common convention is to name inverse trigonometric functions using an arc prefix arcsin(x), arccos(x), arctan(x), etc (This convention is used throughout this article) This notation arises from the following geometric relationships citation needed when measuring in radians, an angle of θ Prove That Tan 1x Tan 1 2x1 X 2 Tan 1 3x X 31 3x 2 Tan 1 3 2x 1 6x Differentiate W R T X In differential calculus, the differentiation of the sin inverse function is written in mathematical form as follows ( 1) d d x ( sin − 1 ( x)) ( 2) d d x ( arcsin ( x)) The differentiation of the inverse sin function with respect to x is equal to the reciprocal of the square root of the subtraction of square of x from one Let me rewrite the proof a bit differently We are required to prove (RTP) that $$2\tan^{1}(y \sqrt{1y^2}) \tan^{1} (y) = \frac{\pi}{2}$$ Proof Plan We will use the formula F first, followed by the identity I`tan^( 1)xtan^( 1)\ (2x)/(1x^2)=pitan^( 1)\ (3xx^3)/(13x^2),(x >0)` সত্য যদি Bihar Inter Results 21 BSEB has released class 12mark sheets, Students can Ex 5 3 10 Find Dy Dx In Y Tan 1 3x X3 1 3x2 Ex 5 3 Example 22 Find I Ex Tan 1 X 1 1 X2 Dx Examples Use of Integration by Parts Calculator For the integration by parts formula, we can use a calculator The steps to use the calculator is as follows Step 1 Start by entering the function in the input field Step 2 Next, click on the "Evaluate the Integral" button to get the output Step 3 The integrated value will be displayed in the{eq}\int\frac{2x^2 1}{ (2x 1)(x^2 1)} dx {/eq} Partial Fraction Decomposition Method An integral is solved using the partial fraction decomposition method (if possible) if it contains aClick here👆to get an answer to your question ️ Prove that 2tan^1x = tan^1 2x1 x^2 I Ff X 2tan 1 X Sin 1 2x 1 X 2 X 1 T H E N F 5 Is Equal To What Is The Integration Of Log 1 X 2 Quora Two numbers r and s sum up to 2 exactly when the average of the two numbers is \frac{1}{2}*2 = 1 You can also see that the midpoint of r and s corresponds to the axis of symmetry of the parabola represented by the quadratic equation y=x^2BxC Example 7 Show that tan1 𝑥 tan1 2𝑥/(1 −𝑥2) = tan1 (3𝑥 − 𝑥3)/(1 − 3𝑥2) Solving LHS tan1 𝑥 tan1 2𝑥/(1 − 𝑥2) = tan1 (𝑥Use the distributive property to multiply y by x 2 1 Add x to both sides Add x to both sides All equations of the form ax^ {2}bxc=0 can be solved using the quadratic formula \frac {b±\sqrt {b^ {2}4ac}} {2a} The quadratic formula gives two solutions, one when ± is addition and one when it is subtraction Prove That 1 2tan 1x Cos 1xsqrt 1 Sqrt 1 X 2 2sqrt 1 X 2 Youtube Notes On A Level P3 Integration Notes 1 For the question, tan(2x)tanx = 1, I divided it by tanx, and got the solution as ( 2n 1) π 6 tan2x = cotx = tan(π 2 − x) So, 2x = nπ π 2 − x So, 3x = ( 2n 1) π 2 But the book solved using the formula of tan(2x), and got the solution as ( 6n ± 1) π 6 I can see that my solution has odd multiples of π / 2, which should be 3 Derivatives of the Inverse Trigonometric Functions by M Bourne Recall from when we first met inverse trigonometric functions " sin1 x" means "find the angle whose sine equals x" Example 1 If x = sin1 025 then by using the calculator, x = 15° We have found the angle whose sine is 025 Inverse Trigonometric Formulas Trigonometry is a part of geometry, where we learn about the relationships between angles and sides of a rightangled triangleIn Class 11 and 12 Maths syllabus, you will come across a list of trigonometry formulas, based on the functions and ratios such as, sin, cos and tanSimilarly, we have learned about inverse trigonometry concepts also Misc 44 Value Fo Tan 1 2x 1 1 X X2 Dx Is Miscellaneous Int Tan 1 2x 1 X 2 Dx The inverse function reverses this operation, taking the ratio of sides as input and returning the angle as output sin − 1 ( b c) = θ cos − 1 ( a c) = θ tan − 1 ( b a) = θ = θ = θ = θ This means the inverse trigonometric functions are useful whenever we Example 13 Solve Tan 1 2x Tan 1 3x Pi 4 Class 12 21 Differentiation And Integration Of Trigonometry Function 9709 If Y 2tan 1 X Sin 1 2x 1 X 2 Then Lt Y Lt Let Tan 1 Y Tan 1 X Tan 1 2x 1 X 2 Where X 1 Sqrt 3 Then A Value Of Y Is 1 3x X 3 1 3x 2 2 3x X 3 1 3x 2 3 3x X 3 1 3x 2 4 3x X 3 1 3x 2 Worked Example Implicit Differentiation Video Khan Academy Inverse Trigonometric Functions General Solution Of Trigonometric Equations Pdf Dwrean Lhpsh Tan 1 X Cot 1 X Pi 2 Arctan X Arccot X Pi 2 Youtube Yorkshiremathstutor Com Wp Content Uploads 19 02 8 Differentiation Answers Pdf Integration Of Tan Inverse X Ex 7 6 13 Chapter 7 Class 12 If Y Tan 1 2x 1 X 2 Then Prove That Dy Dx 2 1 X 2 Ex 2 2 4 Prove 2tan 1 1 2 Tan 1 1 7 Tan 1 31 17 Ex 2 2 Solve Tan 1 X Tan 1 2x 1 X 2 Pi 3 Solving The Differential Equation Dy Dx Tan 2 X Y Youtube Tan 1 2x 1 15x 2 Tan 2 Tan 1 X Tan 2 Arctan X Youtube Range Of Tan 1 2x 1 X 2 Is A Pi 4 Pi 4 B Pi 2 Pi 2 C Pi 2 Pi 4 D Pi 4 Pi 2 Integration Of Inverse Tan 2x Integration By Parts Youtube List Of Trigonometric Identities Wikipedia Differentiate Tan 1 2x 1 X 2 W R T Sin 1 2x 1 X 2 Inverse Trigonometric Functions General Solution Of Trigonometric Equations Pdf Dwrean Lhpsh If Y Tan 1 X Show That 1 X 2 D 2y Dx 2 2x Dy Dx 0 Youtube Ex 7 10 3 Evaluate Integrals Sin 1 2x 1 X2 Dx Ex 7 10 Solve The Equation 2 Tan 1 2x 1 Cos 1 X Madasmaths Com Archive Maths Booklets Standard Topics Trigonometry Trigonometric Inverse Functions Pdf Misc 13 Solve 2 Tan 1 Cos X Tan 1 2 Cosec X Miscellaneous The Derivative Of Tan 1 2x 1 X 2 With Respect To Sin 1 2x 1 X 2 Is Youtube Ex 2 2 5 Simplify Tan 1 Root 1 X2 1 X Chapter 2 Notes On A Level P3 Integration Notes Integral 1 Tan 2 X Sec 2 X Youtube Www3 Nd Edu Apilking Math Work Old exams Exams s13 M final sp13 solution Pdf Solve For X Cos 1 X 2 1 X 2 1 1 2tan 1 2x 1 X 2 2pi 3 Youtube How To Solve Tan 1 2x 1 X2 Cot 1 1 X2 2x Pie 3 Brainly In Draw The Graph Of Y Tan 1 2x 1 X 2 If Tan 1 X2 Y2 X2 Y2 A Prove That Dy Dx X 1 Tana Y 1 Tana Brainly In Prove That Tan 1 X Tan 1 2x 1 X 2 Tan 1 3x X 3 1 3x 2 X Lt 1 Sqrt 3 First Degree Equation 2x 1 0 Lunlun Com Tan 1 X Tan 1 Y Tan 1 X Y 1 Xy Arctan X Arctan Y Arctan X Y 1 Xy Youtube 1 Misc 44 Value Fo Tan 1 2x 1 1 X X2 Dx Is Miscellaneous Prove That Sin 1 2x 1 X 2 2sin 1x X 1 2 What Is Cos Tan 1 X Quora Find The Value Of Tan 1 2 Sin 1 2x 1 X 2 Cos 1 1 Y 2 1 Y 2 X 1 Y 0and X Y 1 Integral Of 1 X 2 9 Substitution Youtube 2tan 1 X Tan 1 2x 1 X 2 2arctan X Arctan 2x 1 X 2 Youtube Let U Sin 1 2x 1 X 2 And V Tan 1 2x 1 X 2 Then D U D V A 1 2 B X C 1 X 2 1 X 2 D 1 Inverse Trigonometric Functions General Solution Of Trigonometric Equations Pdf Dwrean Lhpsh Tan 2x Formula What Is Tan 2x Formula Examples Notes On A Level P3 Integration Notes Derivatives Of Inverse Functions Ppt Download Www Amherst Edu Media View Original Ma11spring10finalanswers Pdf Ex 7 11 8 Evaluate Integral 0 Pi Log 1 Tan X Teachoo Integral Of 1 1 X 2 Youtube Example 13 Solve Tan 1 2x Tan 1 3x Pi 4 Class 12 Differentiate Tan 1 1 X 2 X With Respect To Cos 1 2x 1 X 2 When X 0 Ex 7 6 22 Integrate Sin 1 2x 1 X 2 Teachoo Ex 7 6 Integration Of Tan Inverse X Ex 7 6 13 Chapter 7 Class 12 If Y Tan 1 2 X 1 2 2x 1 Then Dy Dx At X 0 Is Youtube Derivative Of Tan Inverse With Chain Rule Youtube Example 3 Show I Sin 1 2x Root 1 X2 2 Sin 1 X Examples Ex 2 2 13 Inverse Trigonometry Tan 1 2 Sin 1 2x 1 X2 Example 7 Show That Tan 1 X Tan 1 2x 1 X2 Inverse Solve For X Tan 1 2x 1 X 2 Cot 1 1 X 2 2x Pi 3 1ltxlt1 Ex 7 6 22 Integrate Sin 1 2x 1 X 2 Teachoo Ex 7 6 Misc 44 Value Fo Tan 1 2x 1 1 X X2 Dx Is Miscellaneous Ex 5 3 13 Find Dy Dx In Y Cos 1 2x 1 X2 Ncert Ex 5 3 Ex 7 6 22 Integrate Sin 1 2x 1 X 2 Teachoo Ex 7 6 2tan 1 X Sin 1 2x 1 X 2 2arctan X Arcsin 2x 1 X 2 Youtube Inverse Trigonometry Trigonometric Functions Sine Evaluate Inttan 1 X Dx Domain Range Of Inverse Tangent Function Video Khan Academy Differentiate Tan 1 2x 1 X 2 With Respect To Cos 1 1 X 2 1 X 2 If 0 X 1 Solve Tan 1 1 1 2x Tan 1 1 1 4x Tan 1 2 X 2 Ex 2 2 9 Class 12 Inverse Cbse Tan 1 X Root X2 Ex 2 2 5 Simplify Tan 1 Root 1 X2 1 X Chapter 2 Example 13 Solve Tan 1 2x Tan 1 3x Pi 4 Class 12 Sin X 2 Tan X 2 1 Tan 2 X 2 Eraoco Misc 14 Solve Tan 1 1 X 1 X 1 2 Tan 1 X Ncert Derivatives Of Inverse Functions Ppt Download 1 How Do You Find The Equation Of The Tangent Line To The Curve Y X 4 2x 2 X At 1 2 Socratic































































































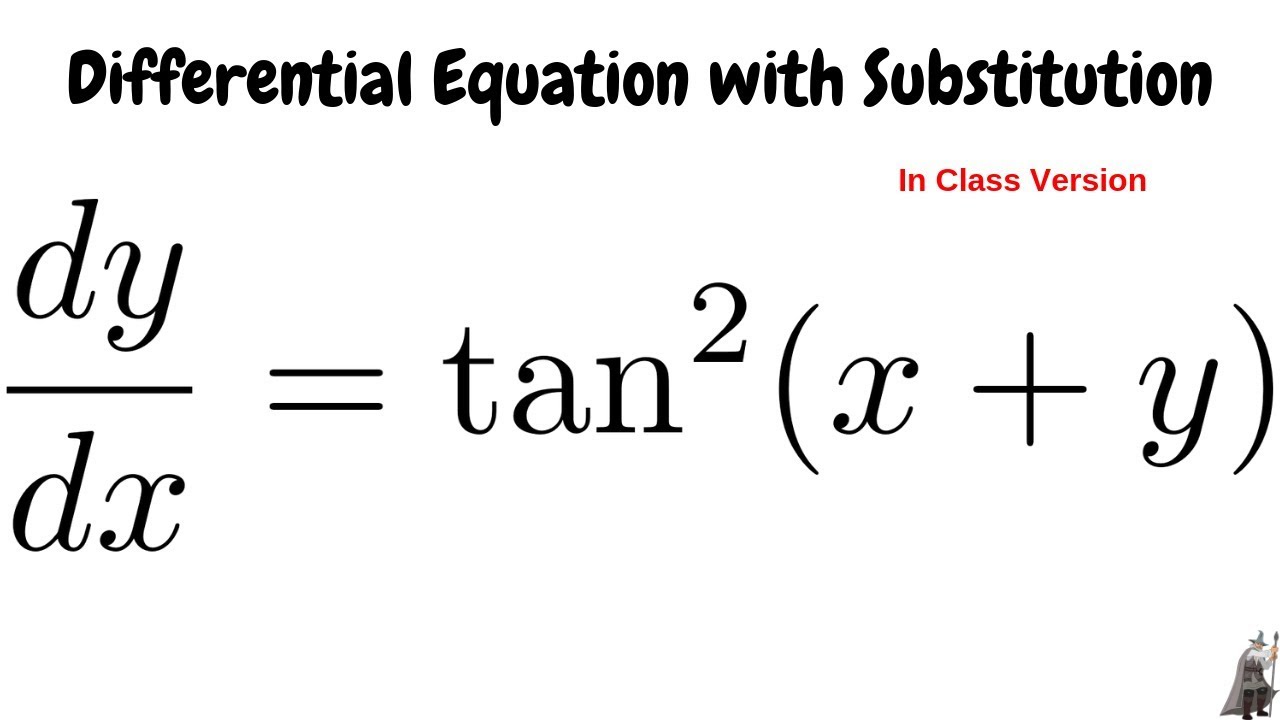

































































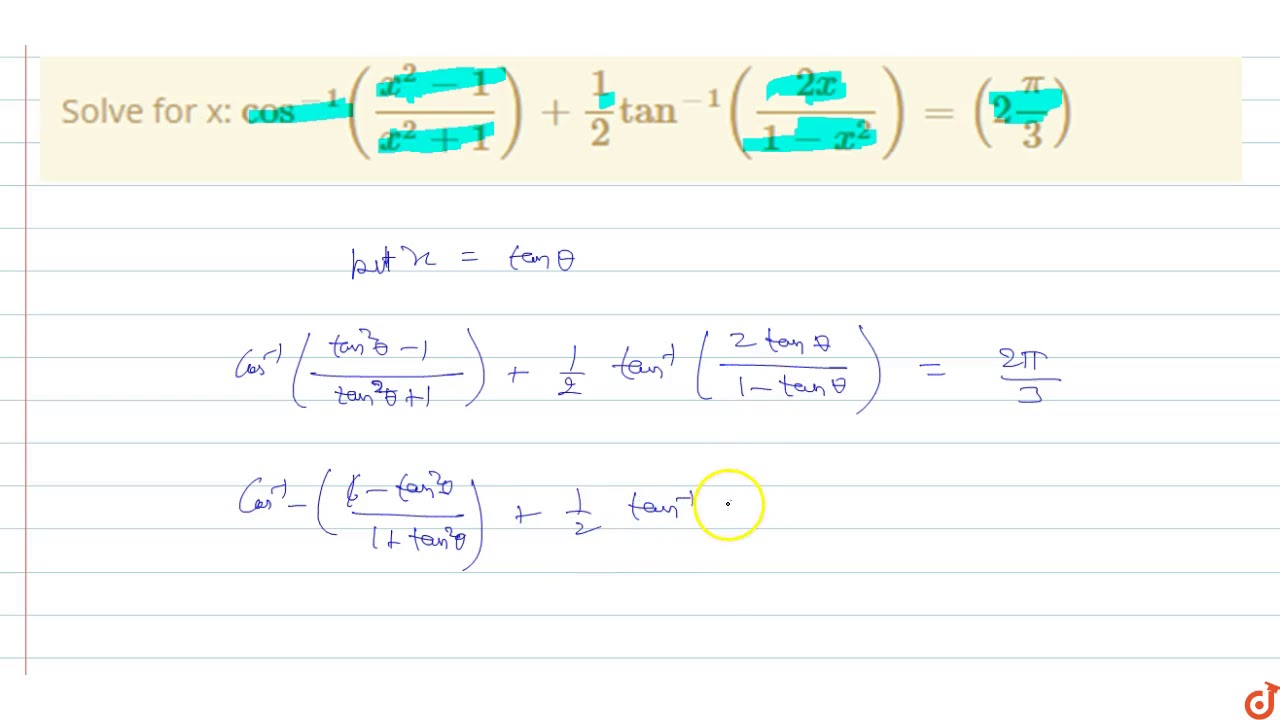





















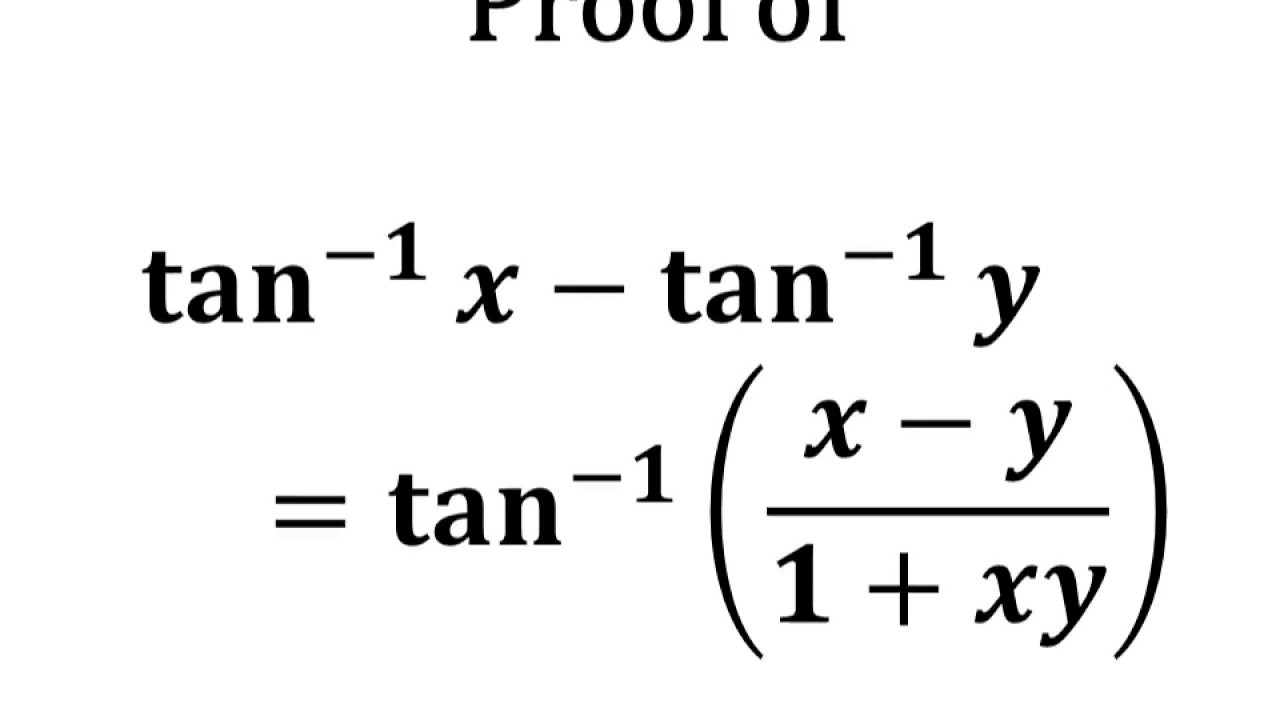





















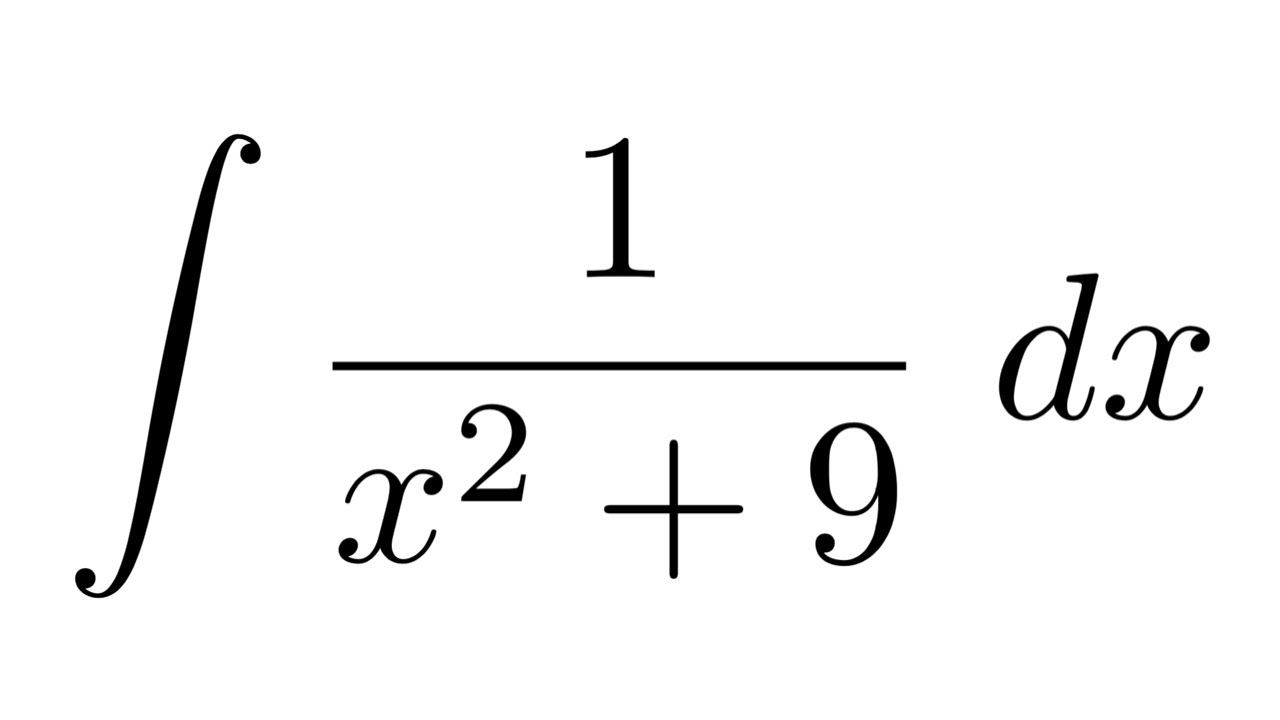



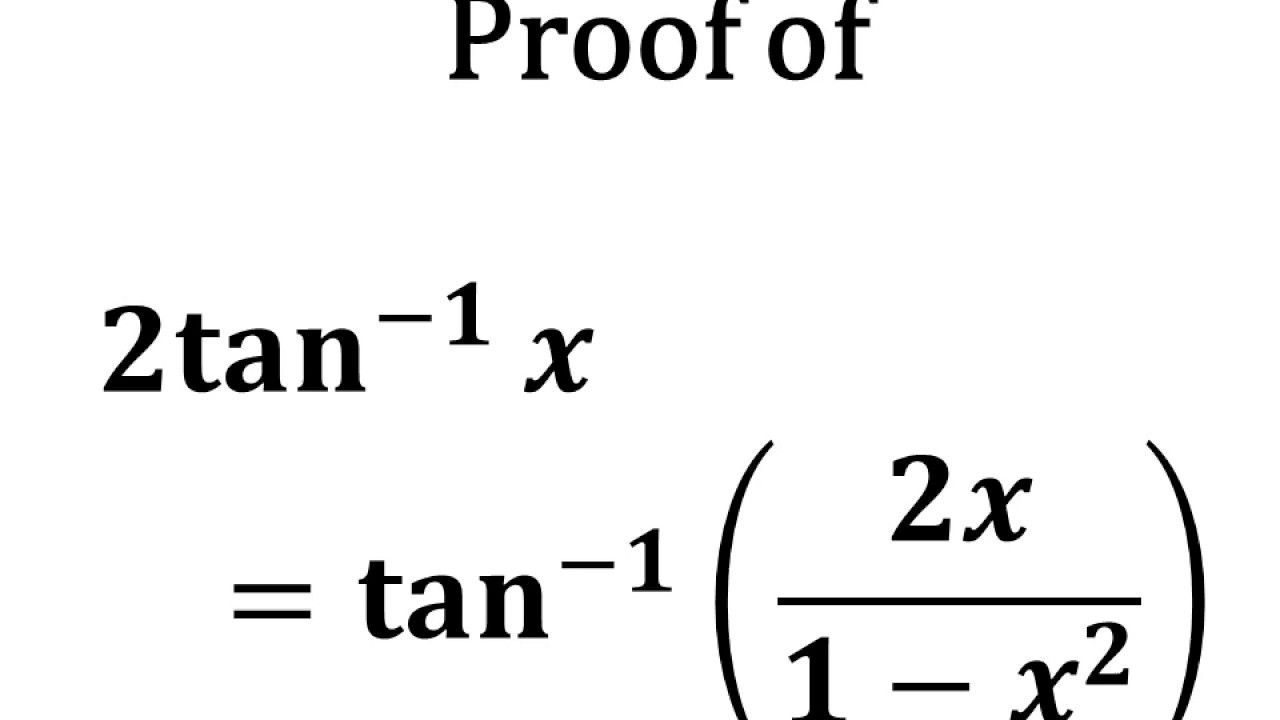







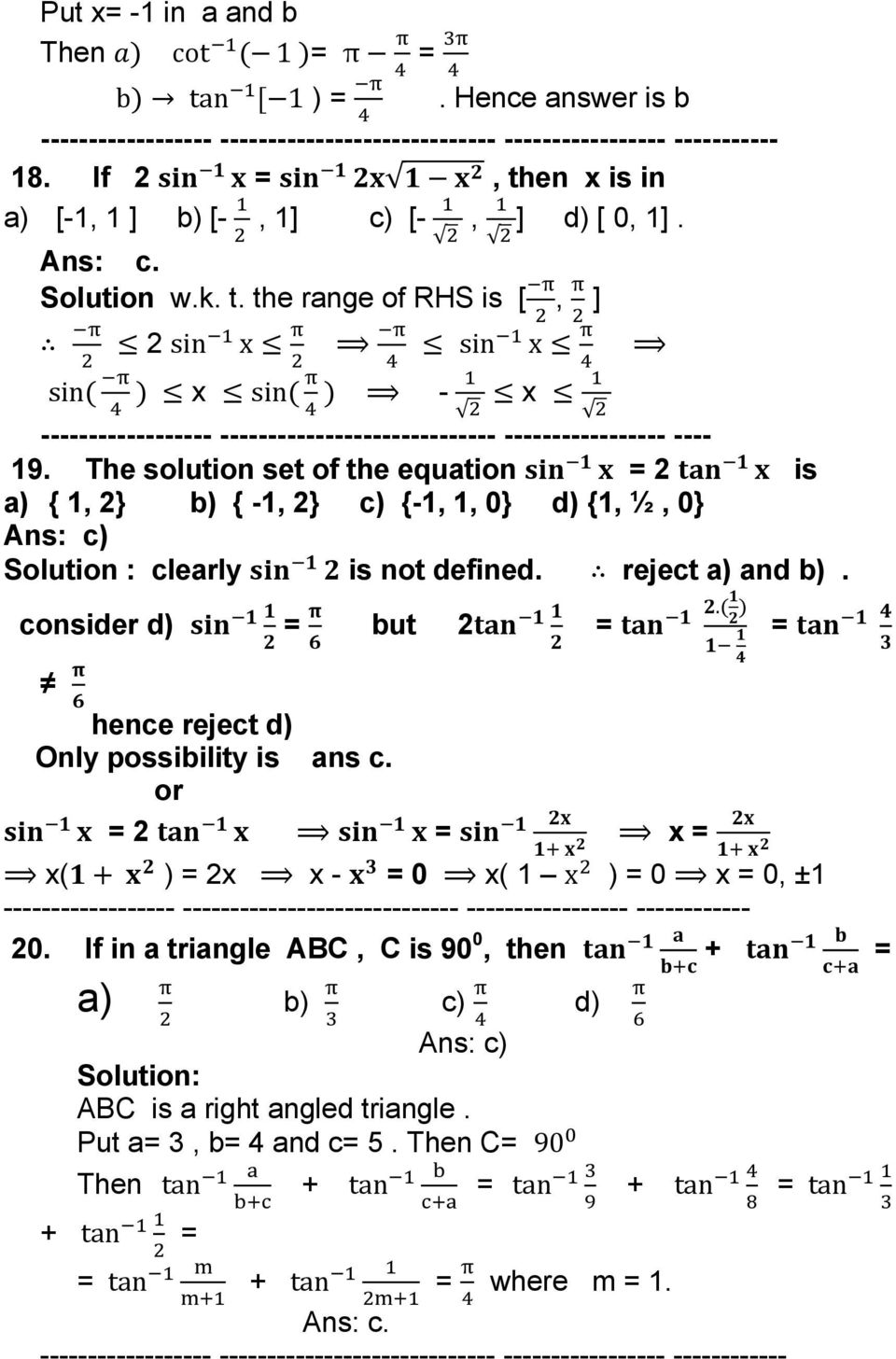











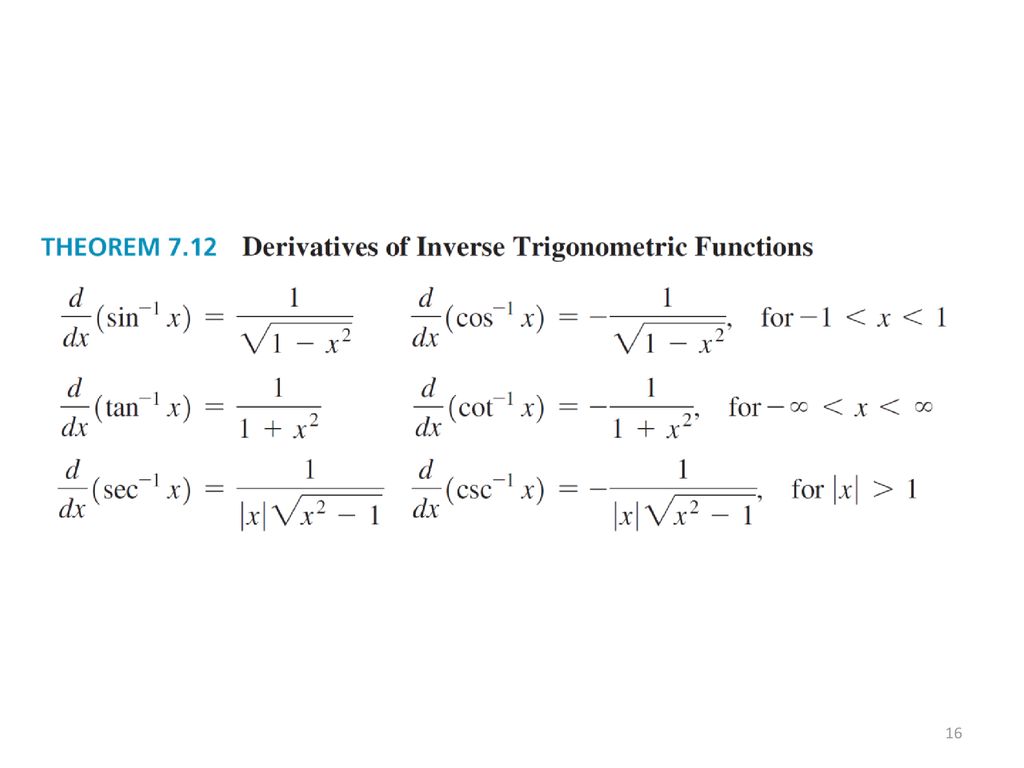














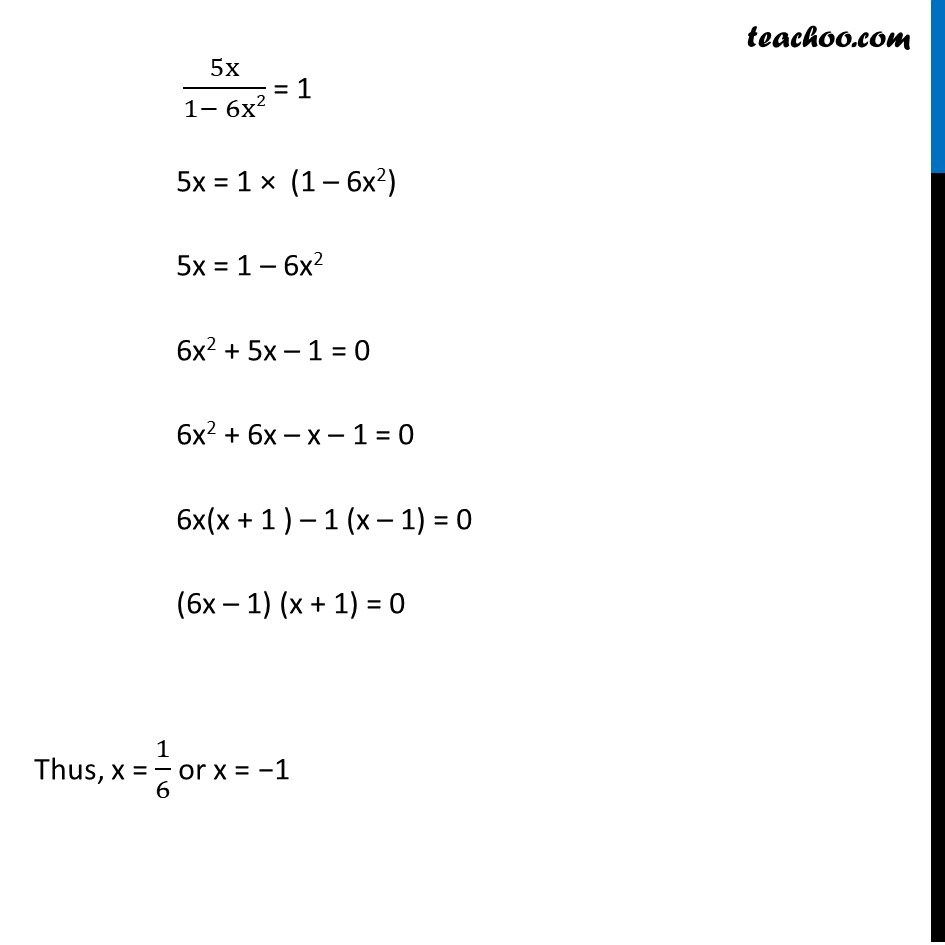







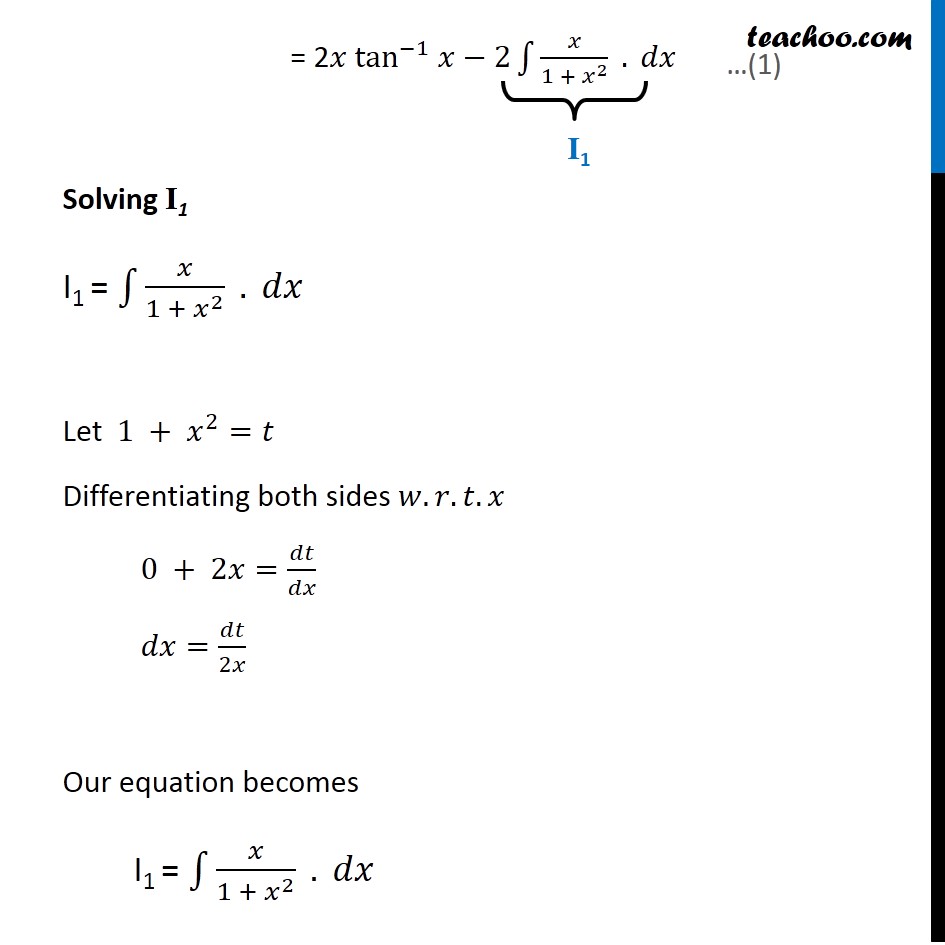



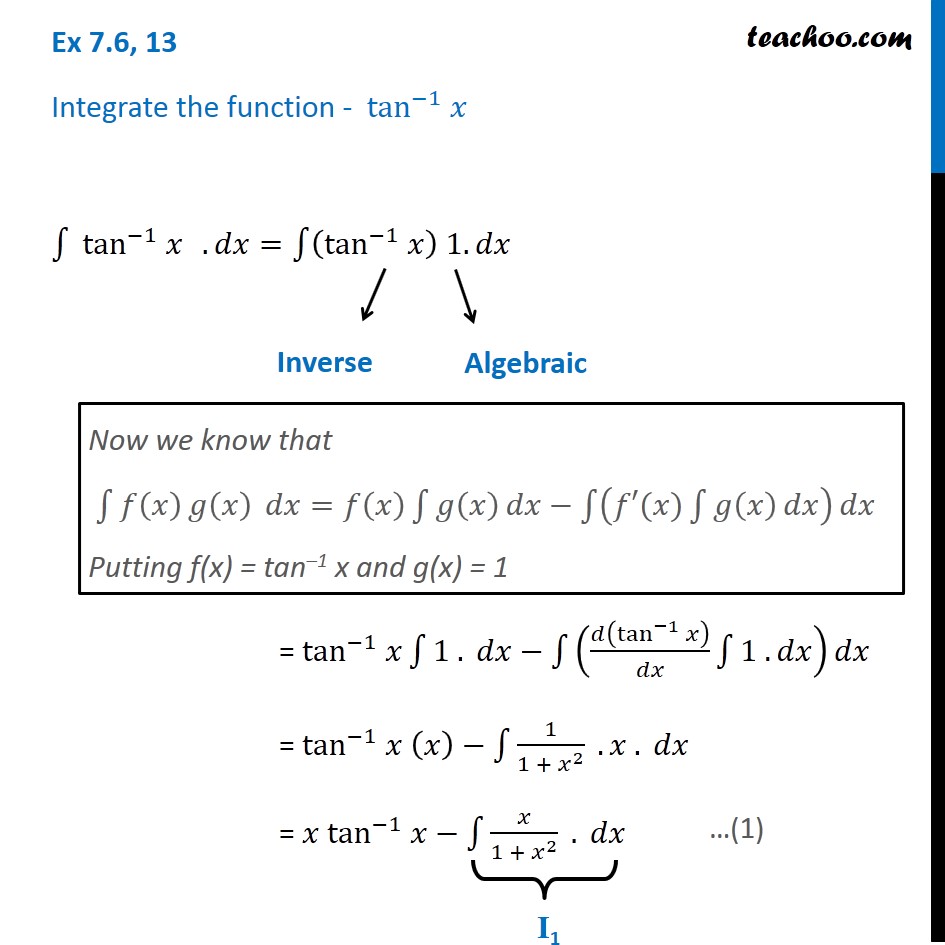




























































































0 件のコメント:
コメントを投稿