1) 1 2) 2 3) 1 4) 4 Answer (2) 2 Solution (cot x – tan x)/ cot 2x = tan 2x (1/tan x) – tan x = tan 2x (1 – tan 2 x)/tan x = tan 2x (1 – tan 2 xThis function has no specific value It cannot be easily simplified Let's say the question was written with an angle value like A instead of x Because we're going to reuse X, and Y tan(A) = Y/X, where Y is the opposite side of a triangle, and XTerms in this set (9) sin^2xcos^2x =1 tan^2x1 =sec^2x cot^2x1 =csc^2x

How Do You Prove The Identities Cosx Secx Sinx Cscx Sec 2x Tan 2x Socratic
Integrate 1+tan^2x/1+cot^2x
Integrate 1+tan^2x/1+cot^2x- Best Answer 1 / 1 tan^2x 1 / 1 cot^2x = 1/sec^2x 1/csc^2x = cos^2x sin^2x = 1 CPhill Post New AnswerVerify the Identity cot (x)^2 (sec (x)^21)=1 cot2 (x) (sec2 (x) − 1) = 1 cot 2 ( x) ( sec 2 ( x) 1) = 1 Start on the left side cot2(x)(sec2(x)−1) cot 2 ( x) ( sec 2 ( x) 1) Apply pythagorean identity cot2(x)tan2(x) cot 2 ( x) tan 2 ( x) Convert to sines and cosines Tap for more steps Write cot ( x) cot ( x) in sines and cosines



Solved K U 1 Differentiate The Following Choose 4 Only Marks A Y Tan 2x 1 Cot 2x B F X Xsin X 1 X C G X Sec Quot 6x Cos Course Hero
The identity $$\frac{\tan(2x)\tan(3x)}{1\tan(2x)\tan(3x)}$$ Stack Exchange Network Stack Exchange network consists of 177 Q&A communities including Stack Overflow , the largest, most trusted online community for developersTan^2x1=sec^2x Solve for tan^2x Tan^2x=sec^2x1 1cot^2x=csc^2x solve for 1 1=csc^2xcot^2x 1cot^2x=csc^2x solve for cot^2x Cot^2x=csc^2x1 RECOMMENDED TEXTBOOKS Geometry for Enjoyment and Challenge New Edition Milauskas, Rhoad, Whipple 256 expertverified explanations Geometry Common Core`(1tanx )^2 (1cotx )^2 ` `= 12tanxtan^2x12cotxcot^2x` `= (1tan^2x)(1cot^2x)2(tanxcotx)` We know that;
Weekly Subscription $199 USD per week until cancelled Monthly Subscription $699 USD per month until cancelled Annual Subscription $3999 USD per year until cancelled User Data Missing Please contact support We want your feedback (optional) (optional) Please add a message Message received Thanks for the feedbackSee the answer (cot^2x1)(sin^2x1)=cot^2x Best Answer 100% (1 rating) Previous question Next question Get more help from Chegg Solve it with our precalculus problem solver and calculatorWhy create a profile on Shaalaacom?
This video shows how to calculate the integral of 1tan^2(x)Now we know that 1/cos 2 (x)= sec 2 (x) So on substitution equation (ii) becomes tan 2 (x) 1= sec 2 (x) Was this answer helpful?RAJKUMAR BAILA Ved Prakash Sharma , former Lecturer at Sbm Inter College, Rishikesh () Answered 8 months ago Author has 99K answers and 6M answer views 1/ (1tan^2 x) 2tan^2 x/ (1tan^2 x) 1/ (cot^2 x) = ?



Http Www Berkeleycitycollege Edu Wp Wjeh Files 12 01 Tri Exercise Identity Pdf




Ppt Analytic Trig Powerpoint Presentation Free Download Id
Solve for x tan1 (2x/1x2) cot1 (1x2/2x) = π/3;1 Given Sin(A) = ⅗ and Cos(B) = 8/17 in Quadrant I, find Sin(AB) a) 24/80 b) 84/85 c) 60/80 d) 60/85 Find Cos(AB) a) 32/80 b) 45/85 c) 13/80 d) 13/85 Find Tan(AB) a) 08 b) 172 c) 421 d) 646 I keep getting an 161 answer but that Algebra 2SOLUTION Verify this identity (tan^2(x)1)/(1tan^2(x)) = 12cos^2(x) I've started a couple different options but none are working out for me Here is what I have so far A) mu Algebra > Trigonometrybasics > SOLUTION Verify this identity (tan^2(x)1)/(1tan^2(x)) = 12cos^2(x) I've started a couple different options but none are working out for me




1 Tan 2x 1 Cos 2x Sin 2x 2sin 4x 1 Sin 2x Trigonometric Identities Mcr3u Youtube



Q Tbn And9gctg1mv4zqwzzx9es1vyuwisok4cikumrujuyo2nyqlvyw5qkqqv Usqp Cau
1 < x < 1 Welcome to Sarthaks eConnect A unique platform where students can interact with teachers/experts/students to get solutions to their queriesClick here👆to get an answer to your question ️ x→0 x^4(cot^4x cot^2x 1)/(tan^4x tan^2x 1) is equal to Join / Login > 11th > Maths > Limits and Derivatives > Limits of Trigonometric Functions`1tan^2x = sec^2x` `1cot^2x = cosec^2x`




Chứng Minh Rằng Tan 2 X Cot 2 X Frac 6 2cos4x 1 Cos4x Cau Hỏi Hoidap247 Com



Solved K U 1 Differentiate The Following Choose 4 Only Marks A Y Tan 2x 1 Cot 2x B F X Xsin X 1 X C G X Sec Quot 6x Cos Course Hero
0 (0) Upvote (0) Choose An Option That Best Describes Your Problem Answer not in Detail Incomplete Answer Answer Incorrect Others Answer not in Detail Incomplete AnswerTan(x y) = (tan x tan y) / (1 tan x tan y) sin(2x) = 2 sin x cos x cos(2x) = cos ^2 (x) sin ^2 (x) = 2 cos ^2 (x) 1 = 1 2 sin ^2 (x) tan(2x) = 2 tan(x) / (1Correct answers 3 question Verify the identity (1 tan^2x)(1 sin^2x) = 1 i know the solution, but not the steps to get me the answers i need i will need to know how to do this on my own for a test soon, so any explanations will be appreciated solution, kinda (1 tan^2x)(1 sin^2x) = sex^2x * cos^2x = 1/cos^2x = 1 here are some identities that i'm pretty sure need to be used, but i'm




Show That 1 1 Tan 2 1 1 Cot 2 1 Sin 2 Sin 4 Brainly In




How Do You Simplify Sec 4x Tan 4x Sec 2x Tan 2x Socratic
= (12tan^2 x)/ (1tan^2 x) tan^2 x , putting tan^2 x = sec^2 x 1 Find an answer to your question 1tan^2x/1cot^2x=(1tanx/1cotx)^2 prove that 1718mlsainipad0im 1718mlsainipad0im Math Secondary School answered 1tan^2x/1cot^2x=(1tanx/1cotx)^2 prove that 2 See answers hello ayushchoubey ayushchoubey Your answer is☝️☝️☝️☝️Question (cot^2x1)(sin^2x1)=cot^2x This problem has been solved!




How Many Can You Derive From First Principles Ppt Download
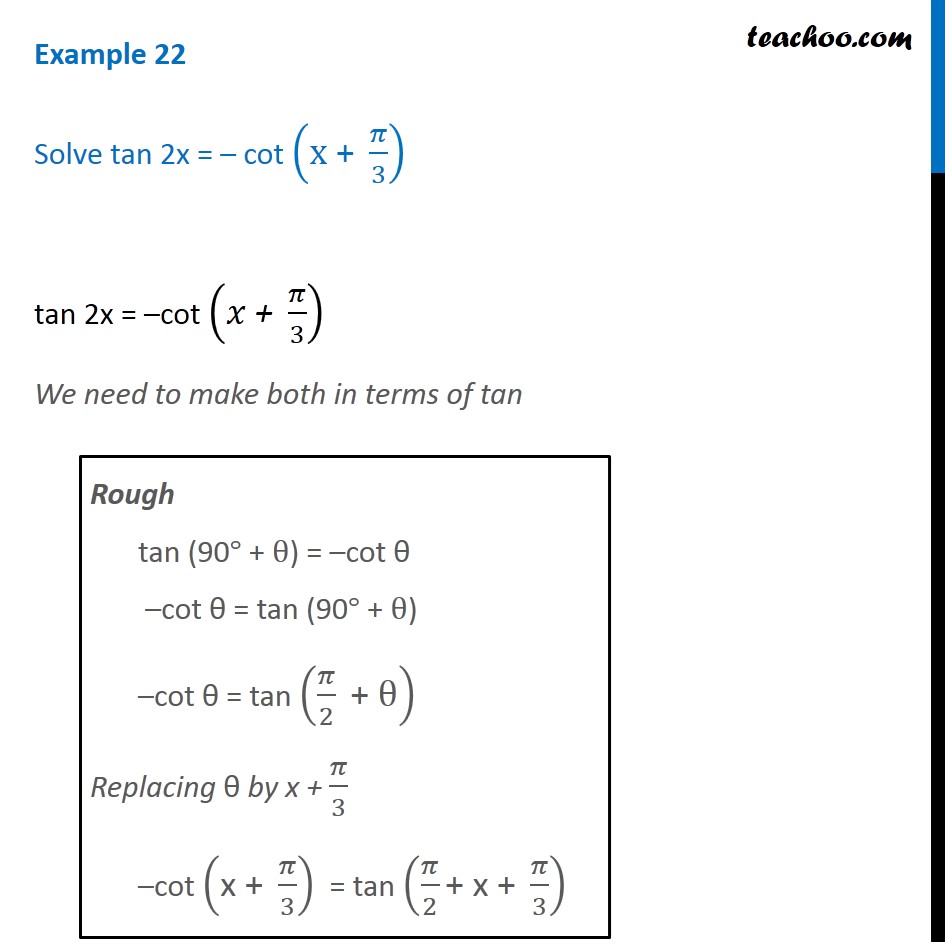



Example 22 Solve Tan 2x Cot X Pi 3 Teachoo Examples
Trigonometria (tan^2x1)/ (tanx)= (cot^2x1)/ (cotx) Si mostri la validità della seguente identità $ (tan^2x1)/ (tanx)= (cot^2x1)/ (cotx)$ E' chiaro che possiamo trasformare il primo membro Category Math Homework Satisfied Customers740 Verified Hi,Welcome to JustAnswer (1tan^2x)/(1cot^2x) = 1sec^2x Substitutetan x = sinx/cosx and cot x = cosx/sinx in the left hand part of the equation(1tan^2x)/(1cot^2x) = 1 (sinx/cosx)^2/ 1 (cosx/sinx)^2= (cos^x sin^x)/cos^2x/(sin^2x cos^2x)/sin^2x= sin^2x/cos^2x= tan^2x eq1Now from the identitytan^2x 1 = sec^2 x tan^2x = 1sec^2x substotute this in eq 1 we get= (1sec^2x )= 1 Welcome to Sarthaks eConnect A unique platform where students can interact with teachers/experts/students to get solutions to their queries Students (upto class 102) preparing for All Government Exams, CBSE Board Exam, ICSE Board Exam, State Board Exam, JEE (MainsAdvance) and NEET can ask questions from any subject and get quick answers by
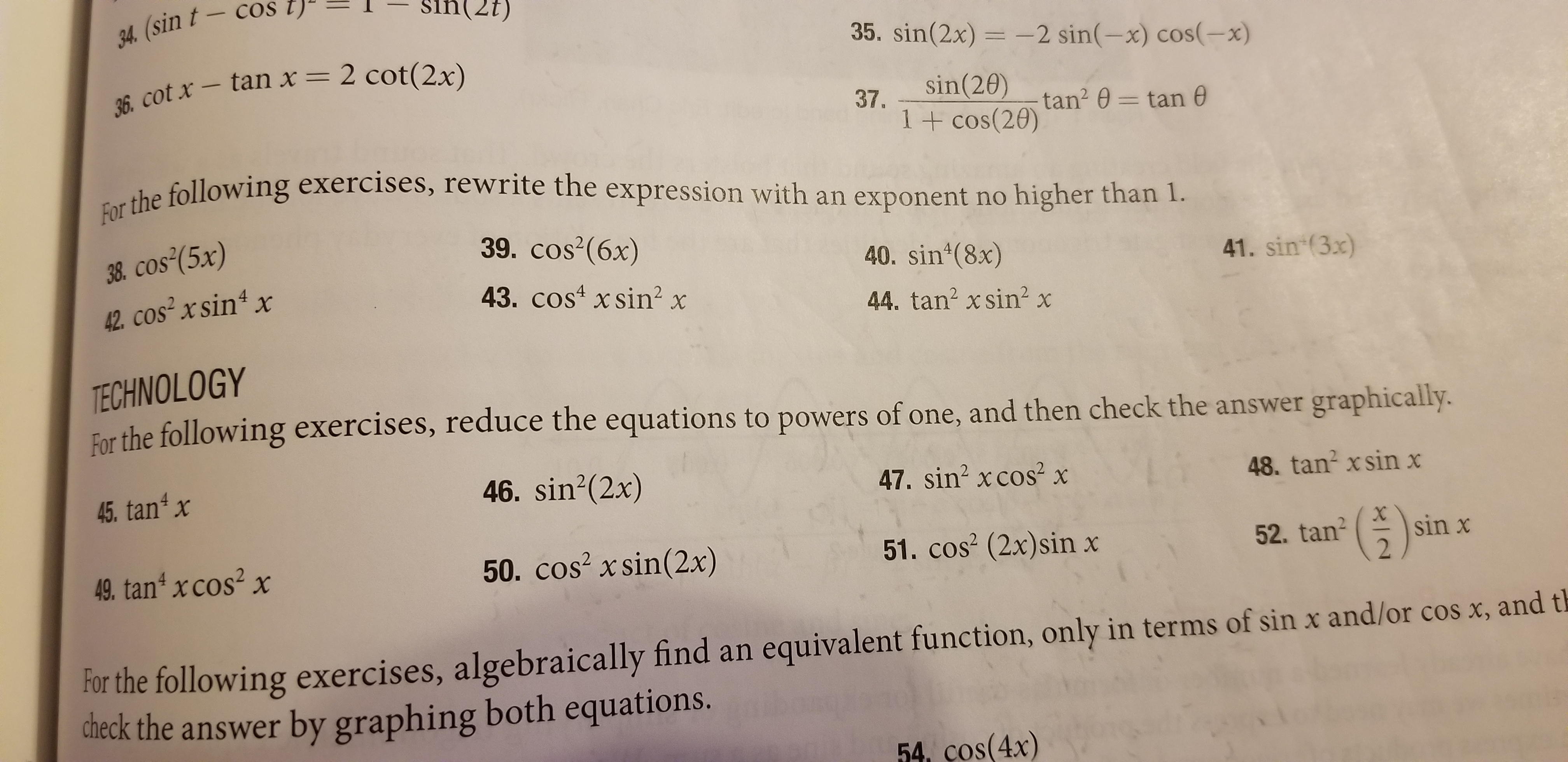



Answered 34 Sin T Cos T 17 Uis 35 Sin 2x Bartleby




Find The Derivative Of The Given Function Y Tan 2x 1 Cot 2x I Tried Converting The Original Function In Terms Of Sin And Cos But It Was Still Too Complicated To Be Called Simplified
Giải phương trình tan^2xcot^2x=1/2 (tanxcotx)1 giúp mink với tan2x cot2x = 1 2 (tanx cotx) 1 t a n 2 x c o t 2 x = 1 2 ( t a n x c o t x) 1 với x ∈ ∈ (0;2π π) tìm số nghiệm pt trên Theo dõi Vi phạm YOMEDIA Toán 11 Chương 1 Bài 3 Trắc nghiệm Toán 11 Chương 1 Consider The Following Equations 1 Cosec 2x Sec 2x Cosec 2xsec 2x 2 Sec 2x Tan 2x Sec 2xtan 2x 3 Cosec 2x Tan 2x Cot 2x Iit 1994 Prove That Sec2x Tan2x Tan Pi 4 X When X Lies Between 0 And Pi 4 Youtube Integral of sec^2x \square!`= 12tanxtan^2x12cotxcot^2x` (1cot^2x)2(tanxcotx)` We know that;Proportionality constants are written within the Get an answer for 'verify (1 tan^2x)/(tan^2x) = csc^2x' and find homework help for other Math questions at eNotes Verify the identity `1/(tan^2x) 1/(cot^2x) = csc^2x




Rd Sharma Solutions For Class 11 Chapter 11 Trigonometric Equations Updated For 21 22 Coolgyan Org



Draw The Graph Of F X Sin X Sqrt 1 Tan 2 X Cos X Sqrt 1 Cot 2 X Then Find The Range Of F X
Simplify (tan (2x))/ (1cot (2x)) tan (2x) 1 − cot(2x) tan ( 2 x) 1 cot ( 2 x) Nothing further can be done with this topic Please check the expression entered or try another topic(1tan^2x)/(1tan^2(x)) 1 = 2cos^2(x)Answers pineapplepizaaaaa Here for a good time of free question carrieaj08 no association stepbystep explanation 1 more answers Mathematics, , WildfireintheHeart




Tan 3x 1 Tan 2x Cot 3x 1 Cot 2xhope It Helps U Welltq Brainly In
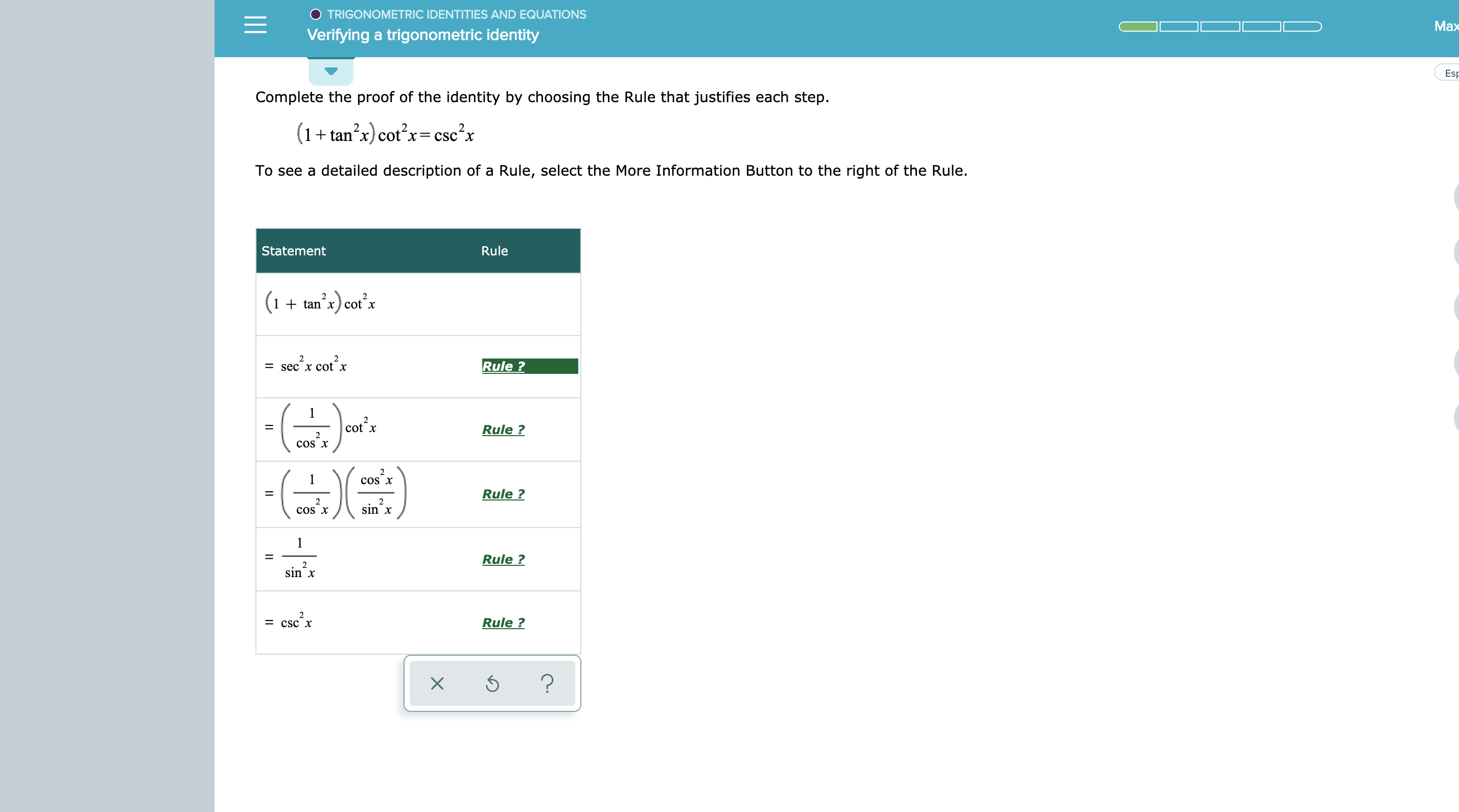



Answered Trigonometric Identities And Equations Bartleby
Answer by nerdybill (7384) ( Show Source ) You can put this solution on YOUR website!Question is solve 1 \cot^2 x = 2 \tan^2 x for x = 0 to 360 degrees I can get 2 solutions which are correct but I am missing the other 2 solutions HFind the derivative of the given function y=(tan 2x)/(1cot 2x), I tried converting the original function in terms of sin and cos but it was still too complicated to be called "simplified" I would really appreciate it if someone could solve for the most simplified form of this derivative



Cpb Us E1 Wpmucdn Com Cobblearning Net Dist 0 6 Files 17 09 Ws Ch 3 Derivative Review 1 Answers Xsp97l Pdf



2
Sin^2x (1cot^2x)=1 distributing the sin^2x sin^2x sin^2xcot^2x = 1 substitute identity of "cot^2x" = cos^2x/sin^2x sin^2x sin^2x (cos^2x/sin^2x) = 1Verify that $$ 2\cos^2x1 = \frac{1\tan^2x}{1\tan^2x}$$ trigonometry Share Cite Follow edited Feb '13 at 2156 Dominic Michaelis 193k 4 4 gold badges 41 41 silver badges 74 74 bronze badges asked Feb 19 '13 at 1538 user user 31 5 5 bronze badges $\endgroup$ 2 4 tan^2x (1tan^2x)/(1cot^2x) 1) First, notice that both the numerator and denominator are Pythagorean Identities Proceed to change them sec^2x/csc^2x 2) Turn into sine and cosine Then multiply (1/cos^2x)/(1/sin^2x) > 1/cos^2x * sin^2x/1 > tan^2x )
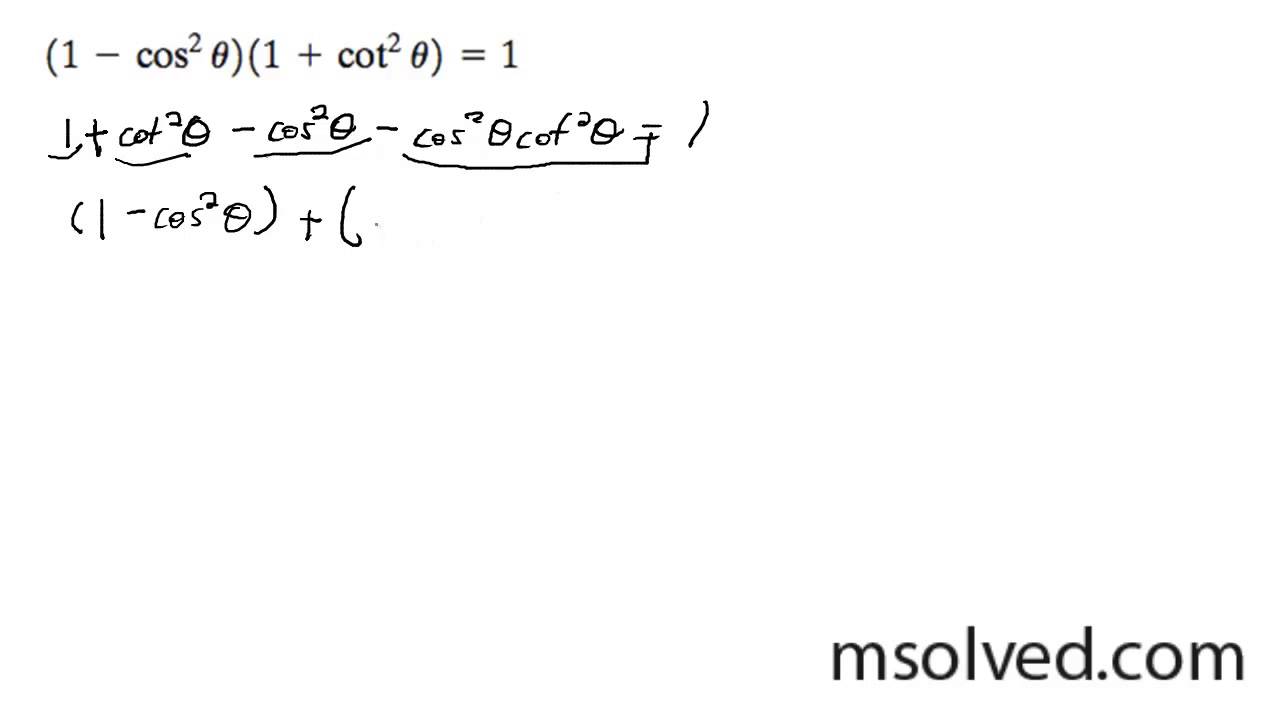



1 Cos 2 1 Cot 2 1 Youtube



Http Scibug Com Public Docs Trigonometry Cheat Sheet Pdf
Answer and Explanation 1 The equation given to us is 2 sec2x−1 − cos2x1 tan2x 2 sec 2 x − 1 − cos 2 x 1 tan 2 x We know the trigonometric identity, sec2θ−tan2θ 1tan^2x/ 1cot^2x = 1sec^2 x Precalculus Circle O below has radius 1 Eight segment lengths are labeled with lowercase letters Six of these equal a trigonometric function of theta Your answer to this problem should be a six letter sequence whose letters represent the1 tan^2(x) = (cos^2(x) sin^2(x))/(cos^2(x)) = sec^2(x) 1 cot^2(x) = (sin^2(x) cos^2(x))/(sin^2(x)) = cosec^2(x) They aren't equal How do you simplify the expression \displaystyle{{\cot}^{{2}}{x}}{1} ?
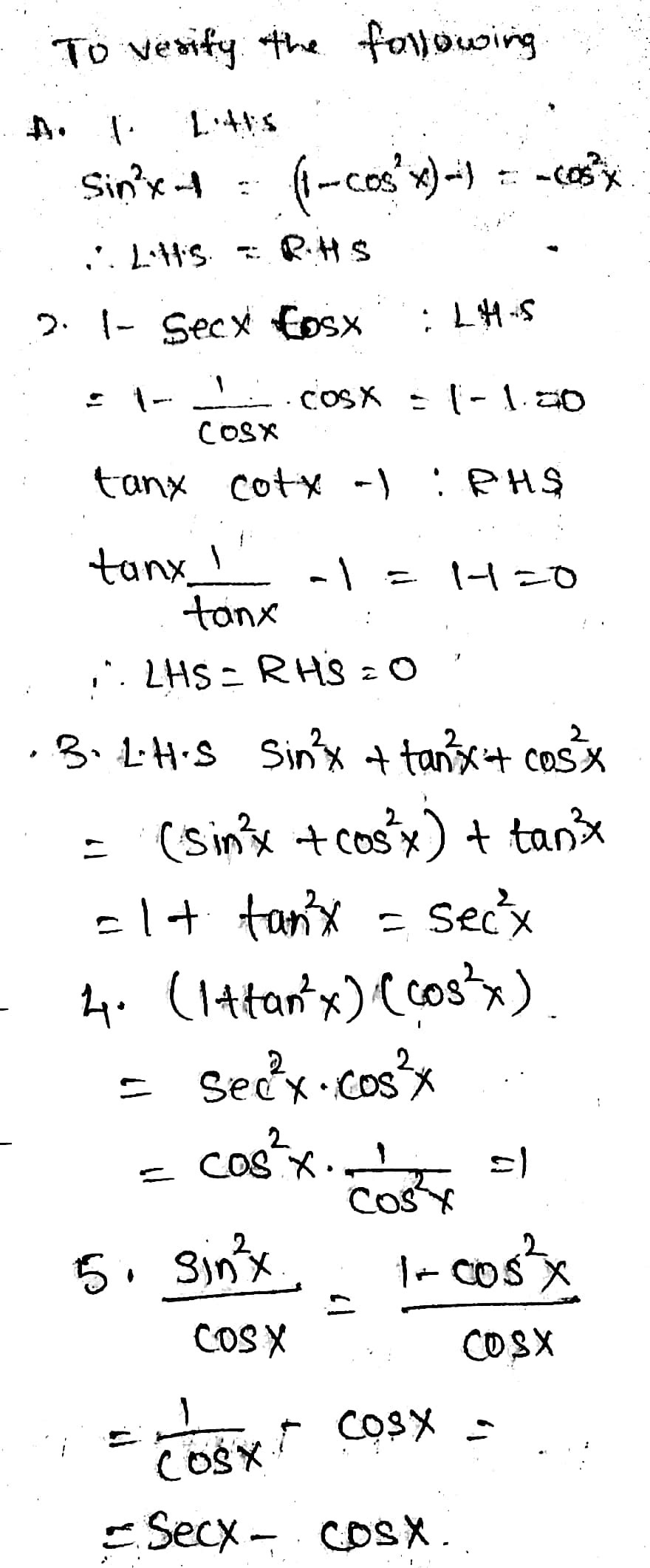



Directions Verify The Following Trigonometric Id Gauthmath
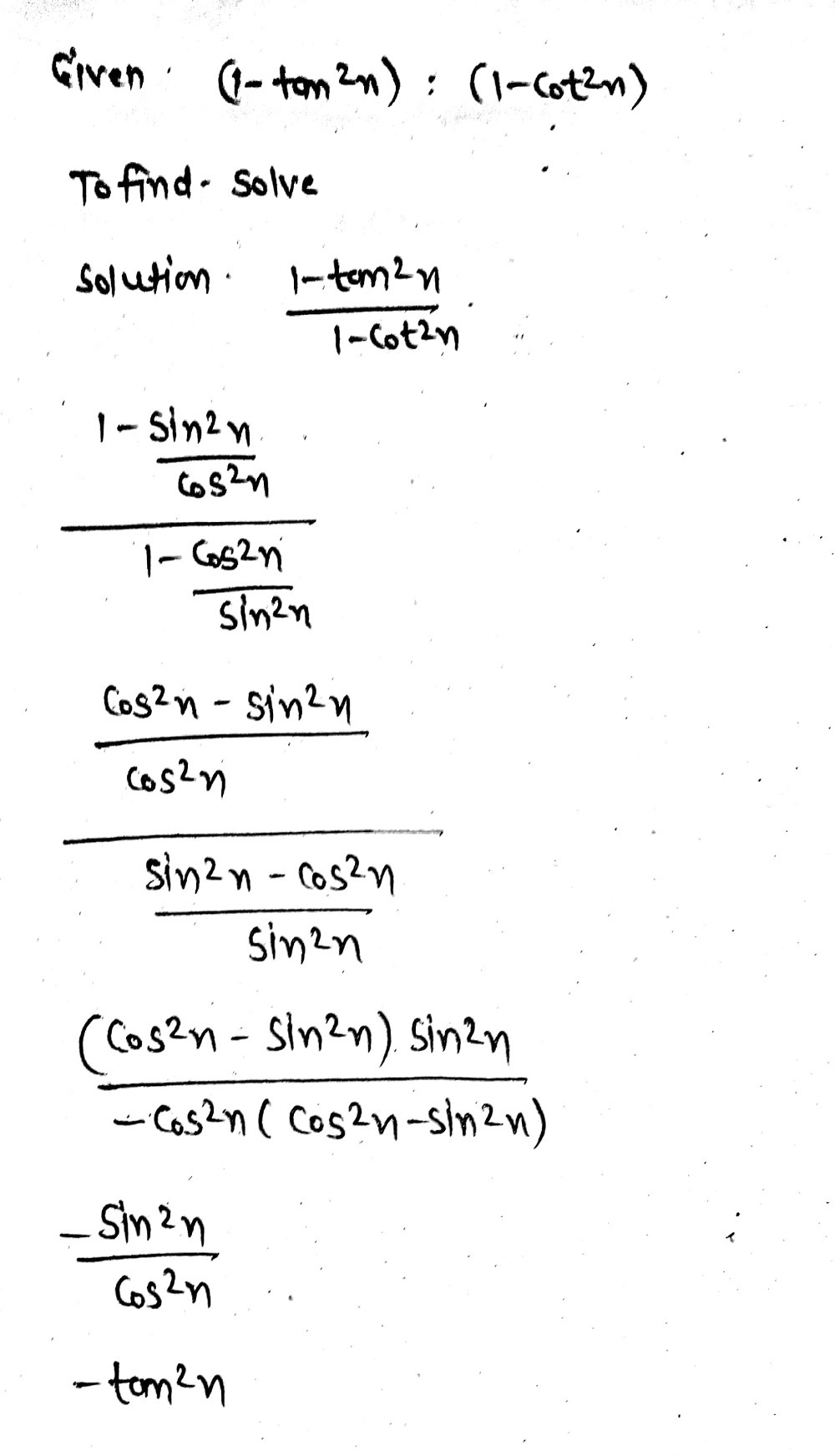



1 Tan 2x 1 Cot 2x Gauthmath
Tanx = t Sec^2 x dx= dt So now it is, 1/(1t)^2 dt This integral is given by 1/1t and t= tanx So, it is cosx/cosx sinx Integral of the function \frac{\cos ^2 x}{1\tan x} Integral of the function 1 Prove that 1tan^2x/1cot^2x=cos^2x1/cos^2x I need to prove this but I don't even know how to start the problemCot^2x(sec^2x1)=1 Simple and best practice solution for cot^2x(sec^2x1)=1 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework If it's not what You are looking for type in the equation solver your own equation and let us solve




1 Sin 2x 1 Cotx Cos 2x 1 Tanx Sinxcosx




Int 1 Tan 2 X 1 Cot 2 X Dx
1 Inform you about time table of exam 2 Inform you about new question papers 3 New video tutorials informationBecause we're going to reuse X, and Y tan (A) = Y/X, where Y is the opposite side of a triangle, and X is the adjacent side Then cot (A) = X/Y = 1/tan (A) So tan^2 (A) cot^2 (A) = (Y/X)^2 (X/Y)^2 = (X^4 Y^4) / (XY)^2 Notice that when X = Y at 45 deg, this function becomes 2 1tan^2x=sec^2x Change to sines and cosines then simplify 1tan^2x=1(sin^2x)/cos^2x =(cos^2xsin^2x)/cos^2x but cos^2xsin^2x=1 we have1tan^2x=1/cos^2x=sec^2x Trigonometry Science The correct identities are 1 tan^2x = sec^2x 1 cot^2x = csc^2x sin^2x cos^2x = 1 which correspond to B and D thank you!!!Tanx = t Sec^2 x dx= dt So now it is, 1/ (1t)^2 dt This integral is given by 1/1t and t= tanx So, it is cosx/cosx sinx tanx = t Sec^2 x dx= dt So now it is, 1/ (1t)^2 dt This integral is given by 1
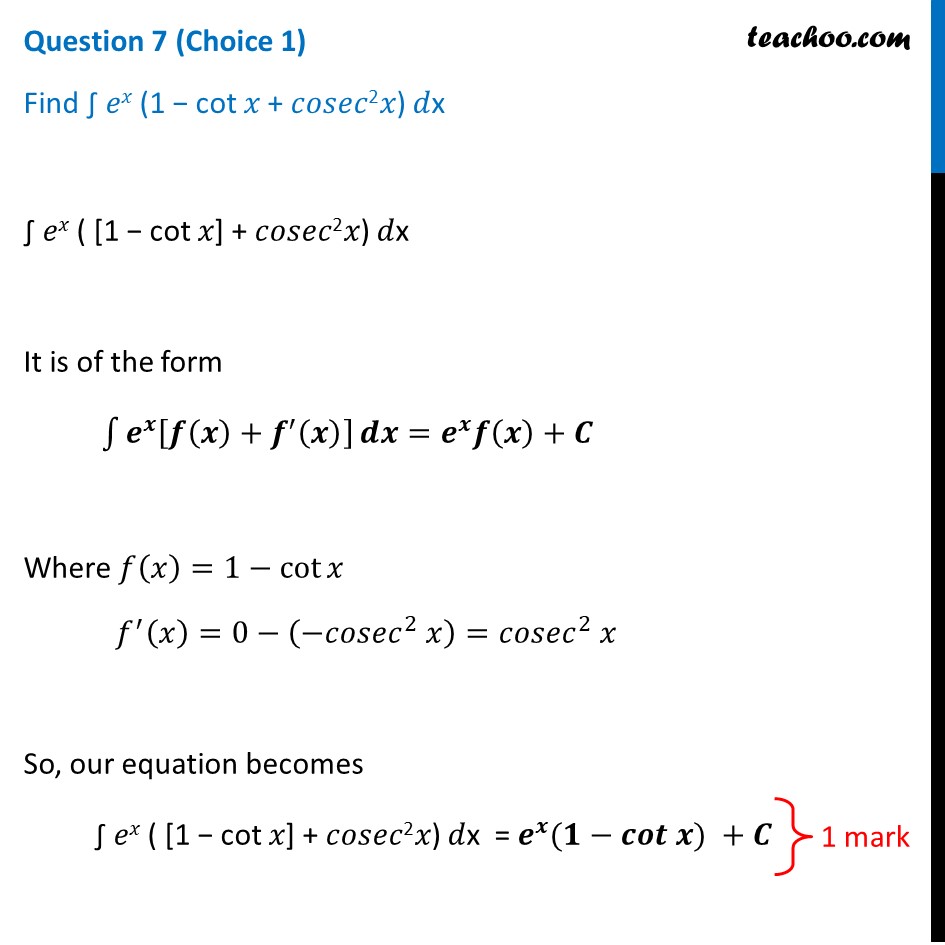



Find E X 1 Cot X Cosec 2 X Dx Integration Video Teachoo



2
Cot^2xtan^2x=1 for all values of x true or false?Click here👆to get an answer to your question ️ The expression (1 tan x tan^2x)(1 cot x cot^2x) has the positive values for x , given byProve \cot(2x)=\frac{1\tan^2(x)}{2\tan(x)} en Related Symbolab blog posts Weekly Subscription $199 USD per week until cancelled Monthly Subscription $699 USD per month until cancelled Annual Subscription $2999 USD per year until cancelled User Data Missing Please contact support




Math22 1reviewerderivativetrigonometricfunction Pdf Trigonometric Functions Mechanics



2
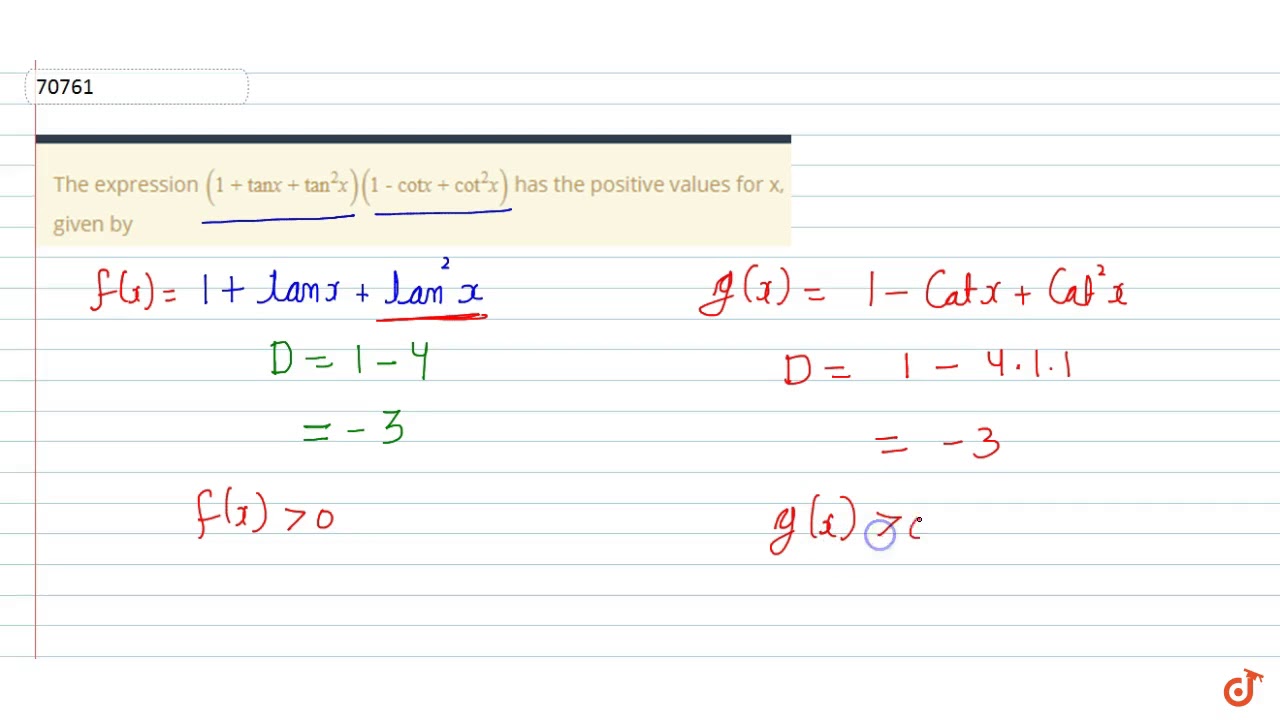



The Expression 1 Tan X Tan 2 X 1 Cot X Cot 2 X Has The Positive Values For X Given Youtube
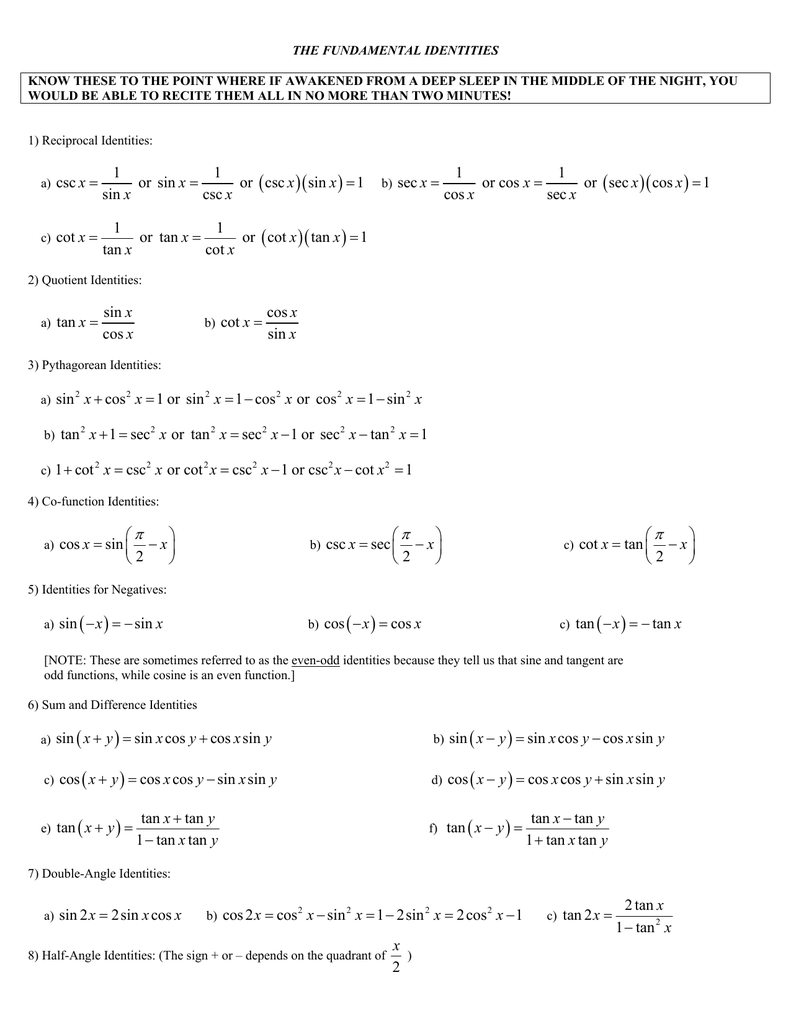



1 1 Csc Or Sin Or Csc Sin 1 Sin Csc X X X X X X 1 1 Sec Or Cos Or




How Do You Prove The Identities Cosx Secx Sinx Cscx Sec 2x Tan 2x Socratic




Integral Of Tan 2x Cot 2x 2 Calculus 1 Trig Integrals Calculus Mathematics Email Subject Lines



Trig Identities Ppt Download




Verifying A Trigonometric Identity Cos X 1 Tan 2 X Cos X Sec X 1 Youtube




Frac Sin 2x 1 Cot 2x Mathit Cotx Snapsolve



2




How Do You Verify 4tan 4 Tan 2x 3 Sec 2x 4tan 2x 3 Kinda Hard Please With All The Steps Thanks Socratic




1 Cot 2x Tan 2x 1 Nedir Acaba Eodev Com
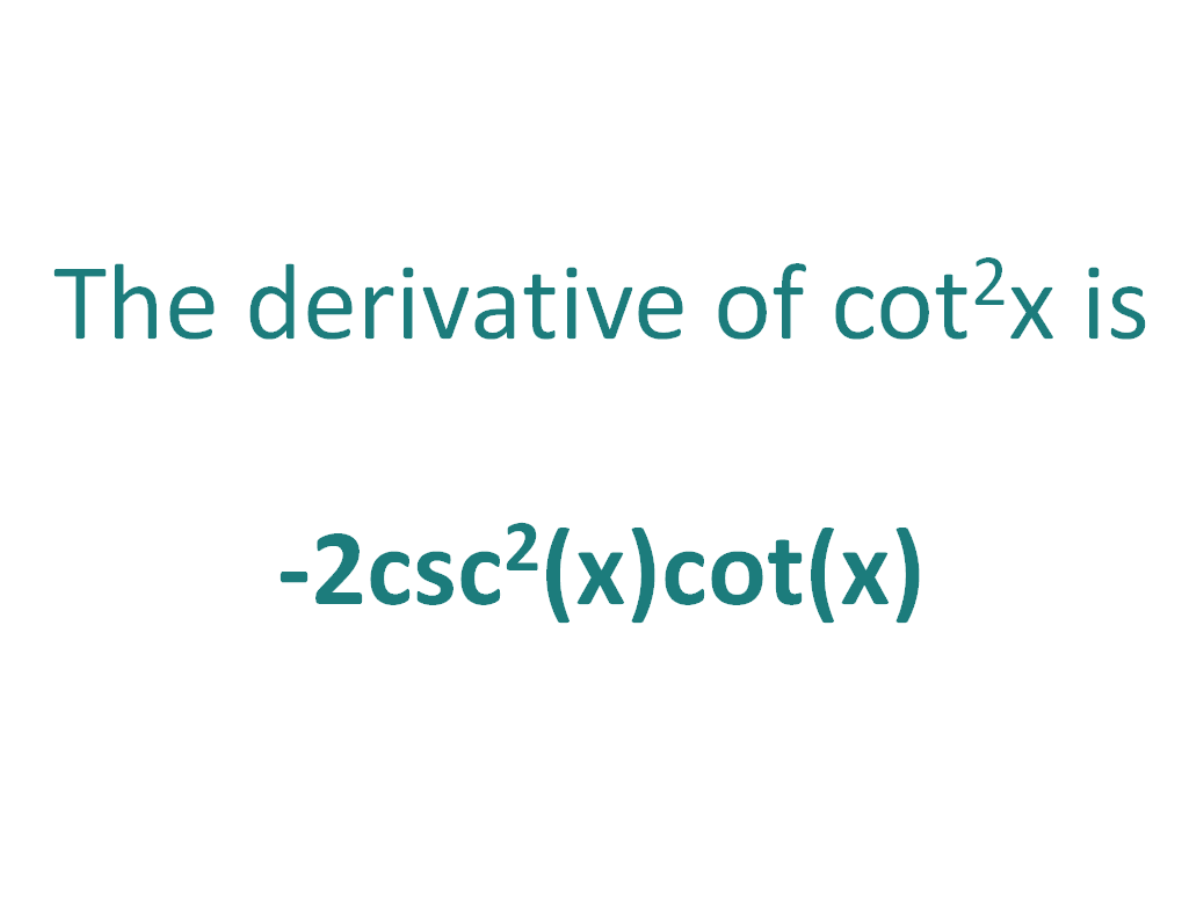



The Derivative Of Cot 2x Derivativeit
.JPG)



Every Day I M Calculatin I D3 Unit Q Pythagorean Identities
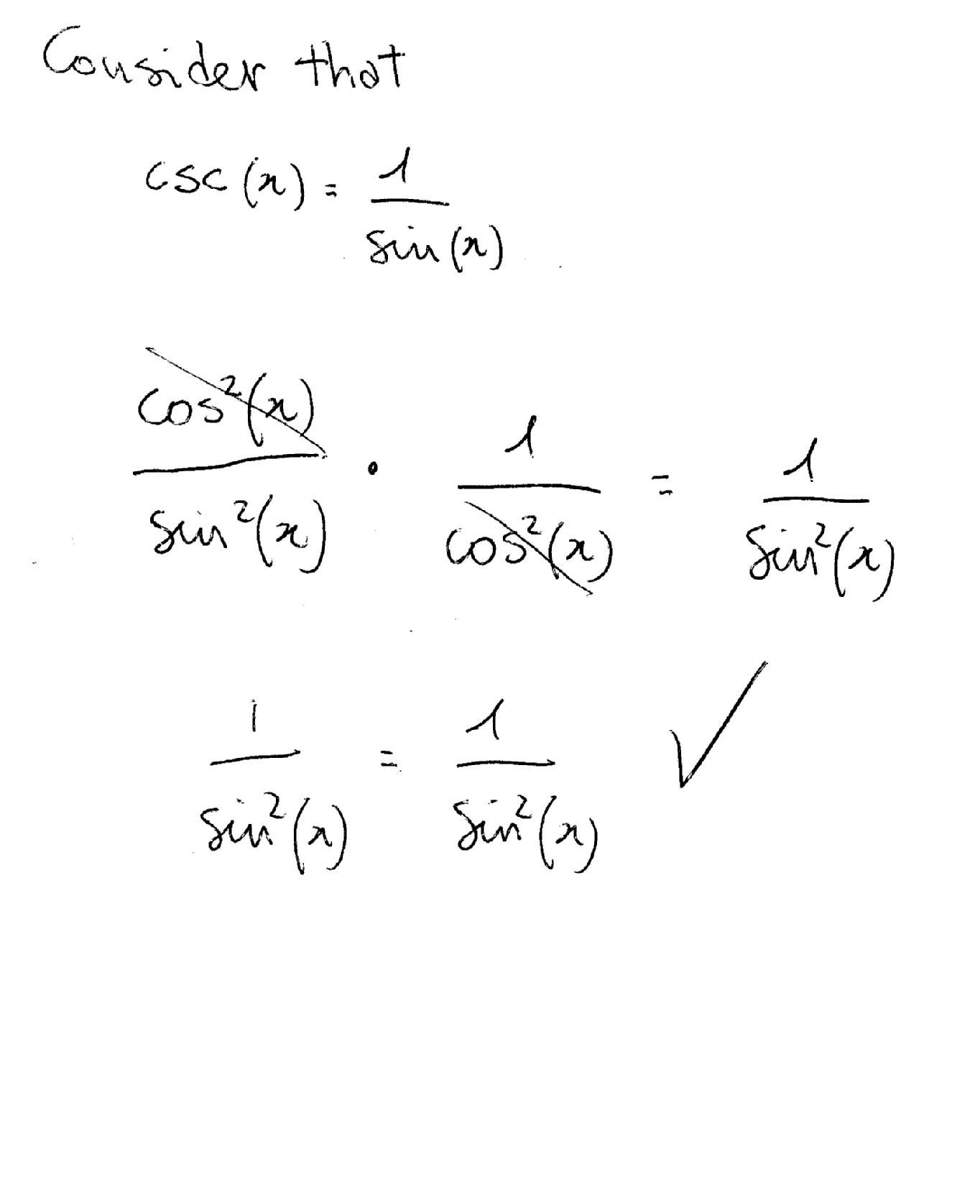



How Do You Verify Cos 2x Sin 2x 1 Cos 2x Csc 2x Socratic




Tan 2x Csc 2x Tan 2x 1 Problem Solving Solving Identity




Prove That I Sin 2x 1 Cos 2x Cot X Ii 1
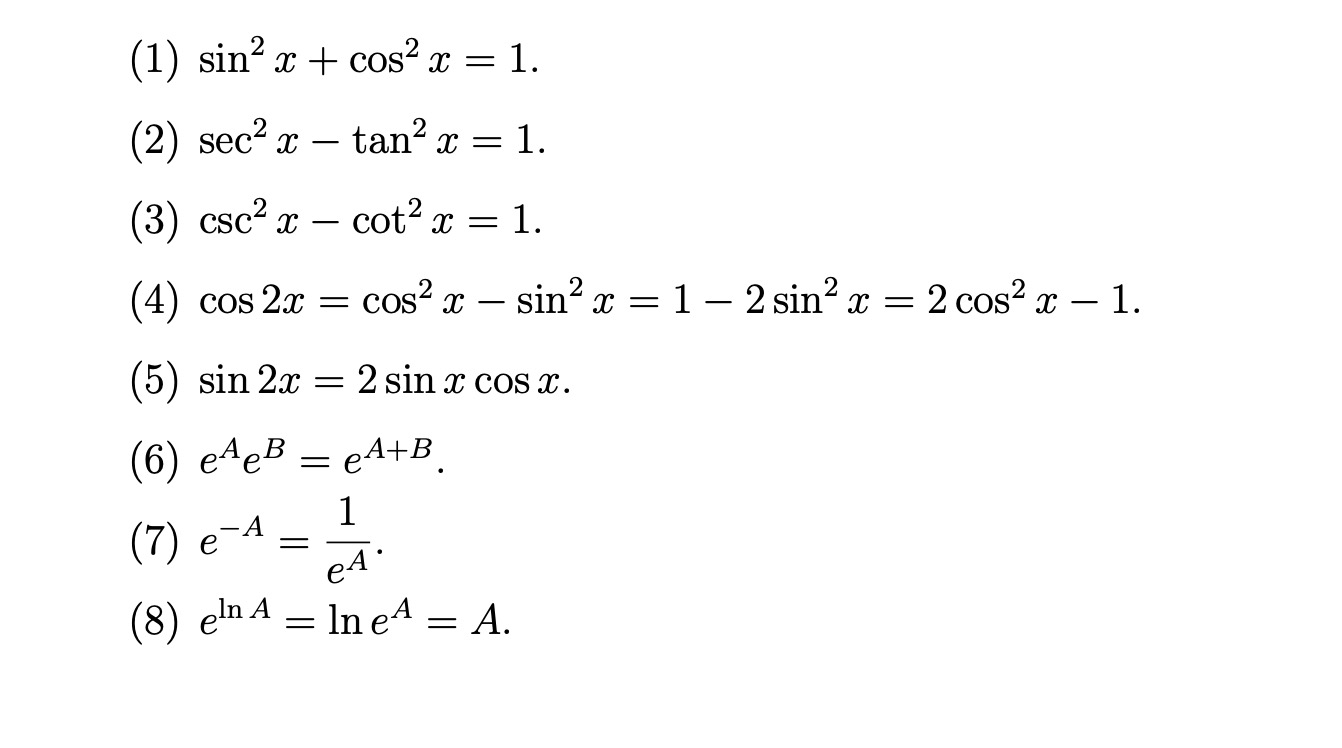



Here Is A List Of Identities Involving Trigonometric Chegg Com
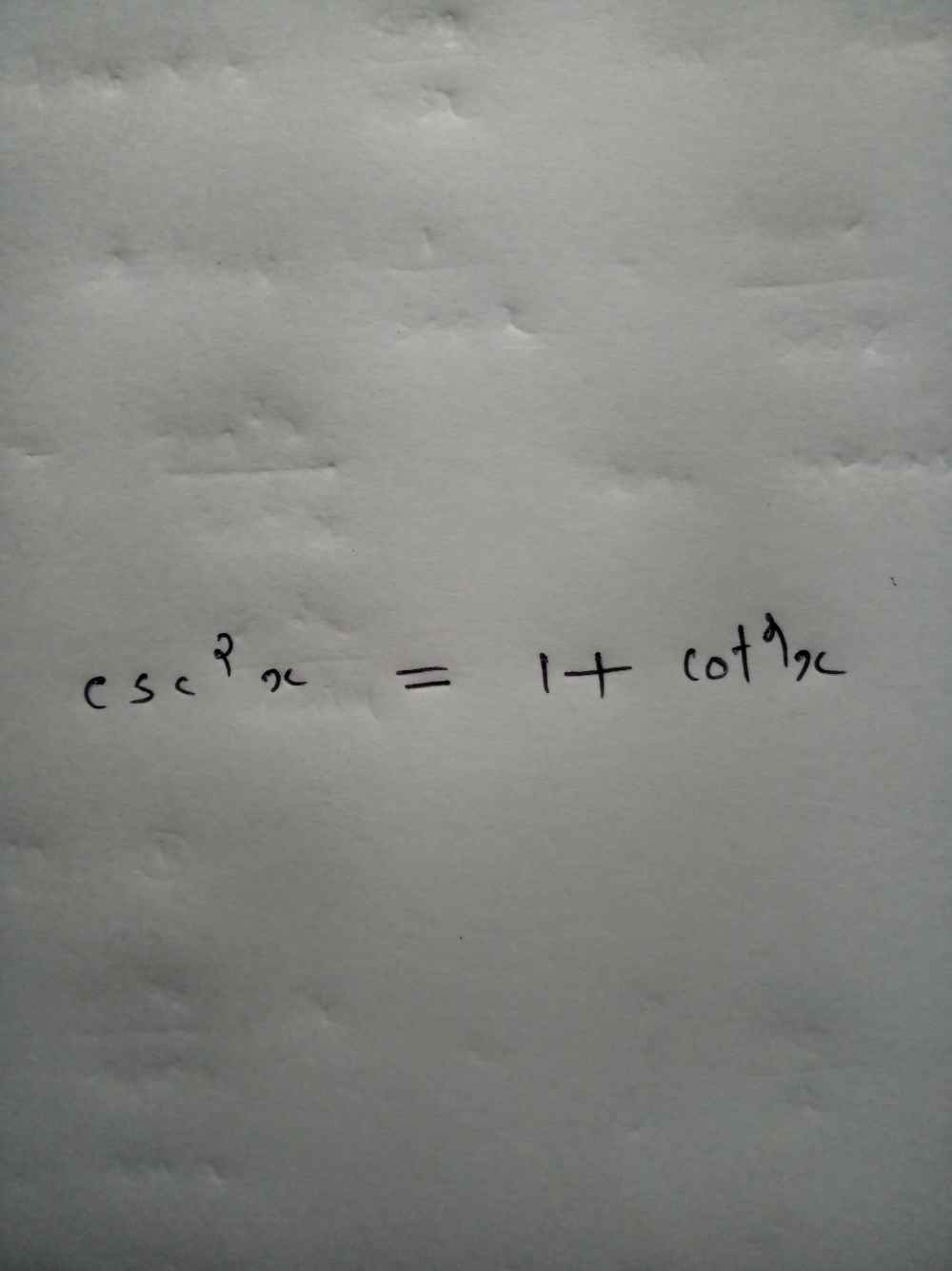



Csc 2x S Select One 1 Sin 2x 1 Sin 2x 1 Tan 2 Gauthmath



Http Www Mpsaz Org Mtnview Staff Clpavlovic Collegealgebra Resources Files Assignment 6 3 Day 1 3 Worksheet Key Pdf




Tan 3x 1 Tan 2x Cot 3x 1 Cot 2x Secx Cosecx 2sincosx Brainly In




Sinx Sinytan Bar2 C0sright See How To Solve It At Qanda




Solve Tan 2 X 1 0 Yahoo Answers Noha Matthieu Lire Un Livre



Www2 Math Binghamton Edu Lib Exe Fetch Php People Mckenzie Trig Identities Worksheet With Answers 2 Pdf



1 If Fx Sin 2x Cos 2x Sec 2x Csc 2x Tan 2x Cot 2x Gauthmath



2



3




Homework 10 1 Sin2 2x 4 Sin2 X Cos2 X Csc 2 Sin Chegg Com




Trig Identity Tan X 1 Tan 2 X Sec X Cos X Csc X Sin X Proved From Both Sides Youtube



1




Answered Power Reducing Formulas Cos 2x 1 Cos Bartleby



How To Prove The Identity In Tanx Cotx 2 Sin2x Quora




How Do You Verify Cot 2x Sec 2x Csc 2x Socratic



Http Www Math Brown Edu Tomg Formulas Pdf




Integration Trig Identities Ppt Download
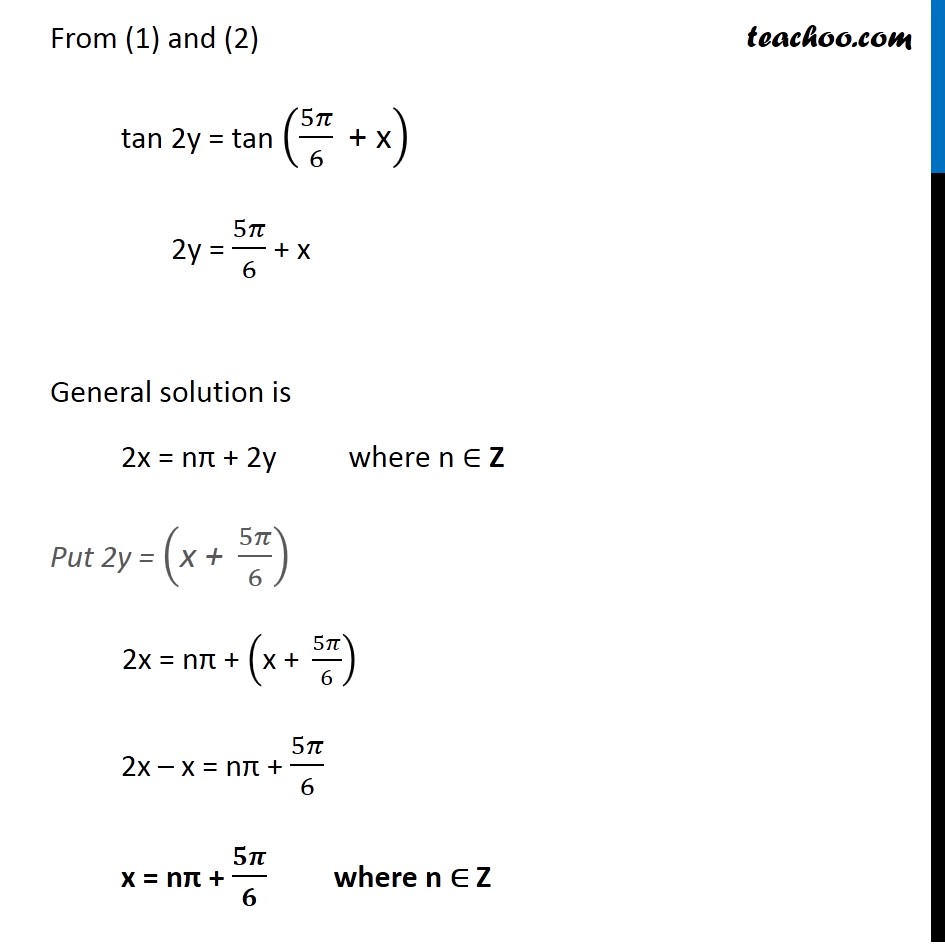



Example 22 Solve Tan 2x Cot X Pi 3 Teachoo Examples




How Do You Prove The Identity Tan 2x Secx 1 1 Cosx Cosx Socratic




Answered 8 In Parts A To E Simplify The Bartleby



Cot X Cos 2 X Tan X Sin 2 X 2 Cot 2x Youtube




Cosec 2x Cot 2x Cot X Youtube



C3 Trig The Student Room
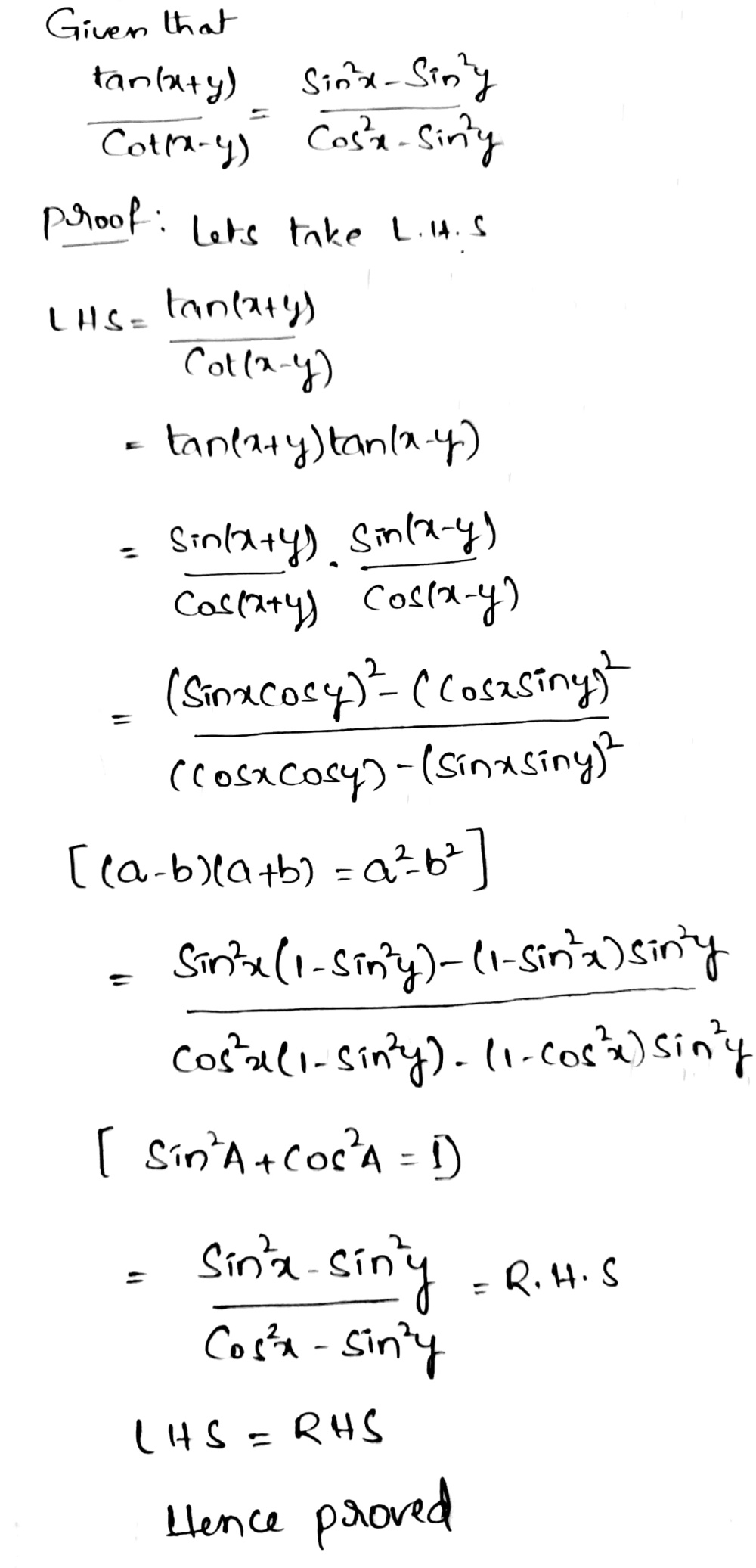



Prove Each Of The Following Frac Tan X Y Cot X Y Frac Sin 2x Sin 2y Cos 2x Sin 2y Frac Tan 2x Tan 2y 1 Tan 2xtan 2y Snapsolve
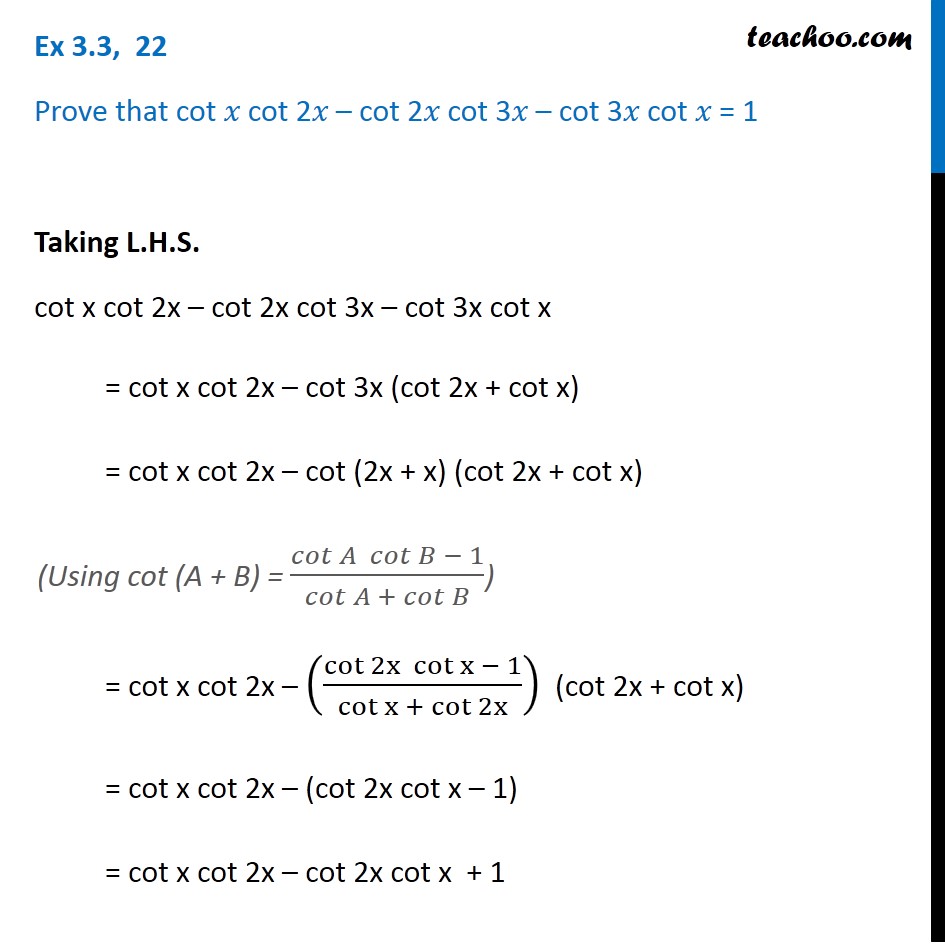



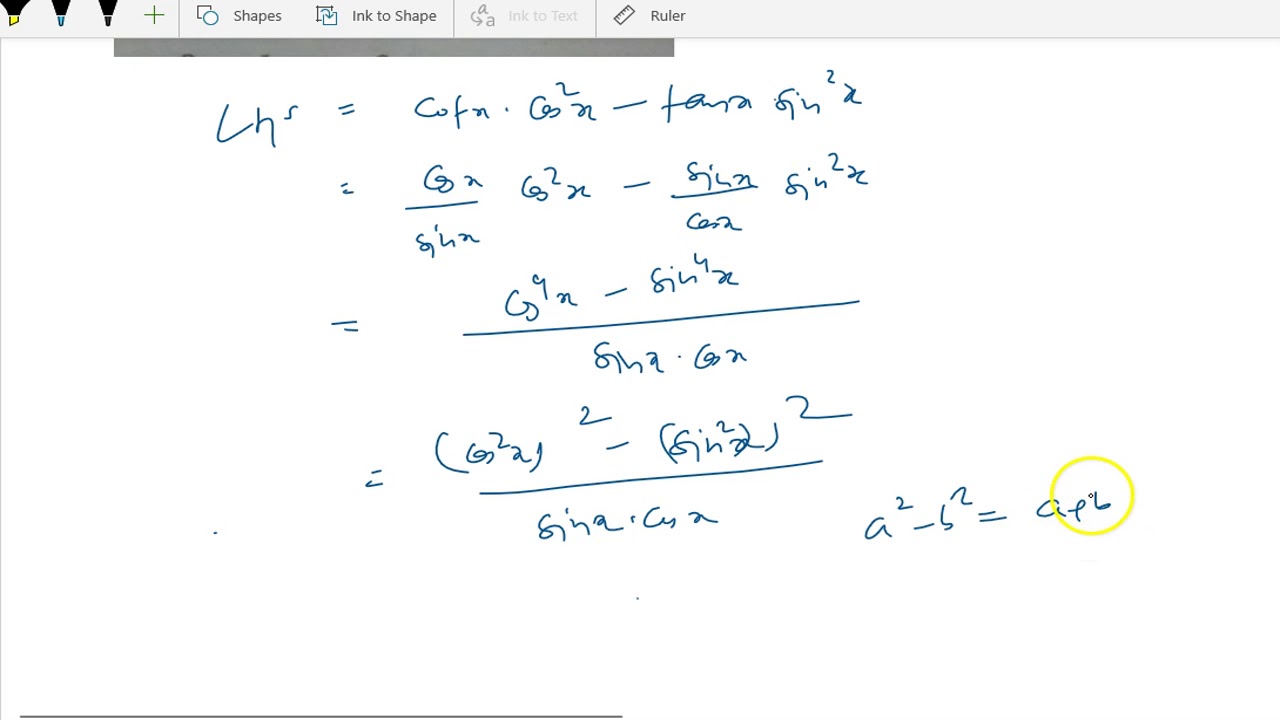
Ex 3 3 22 Prove Cot X Cot 2x Cot 2x Cot 3x Cot 3x Cot X
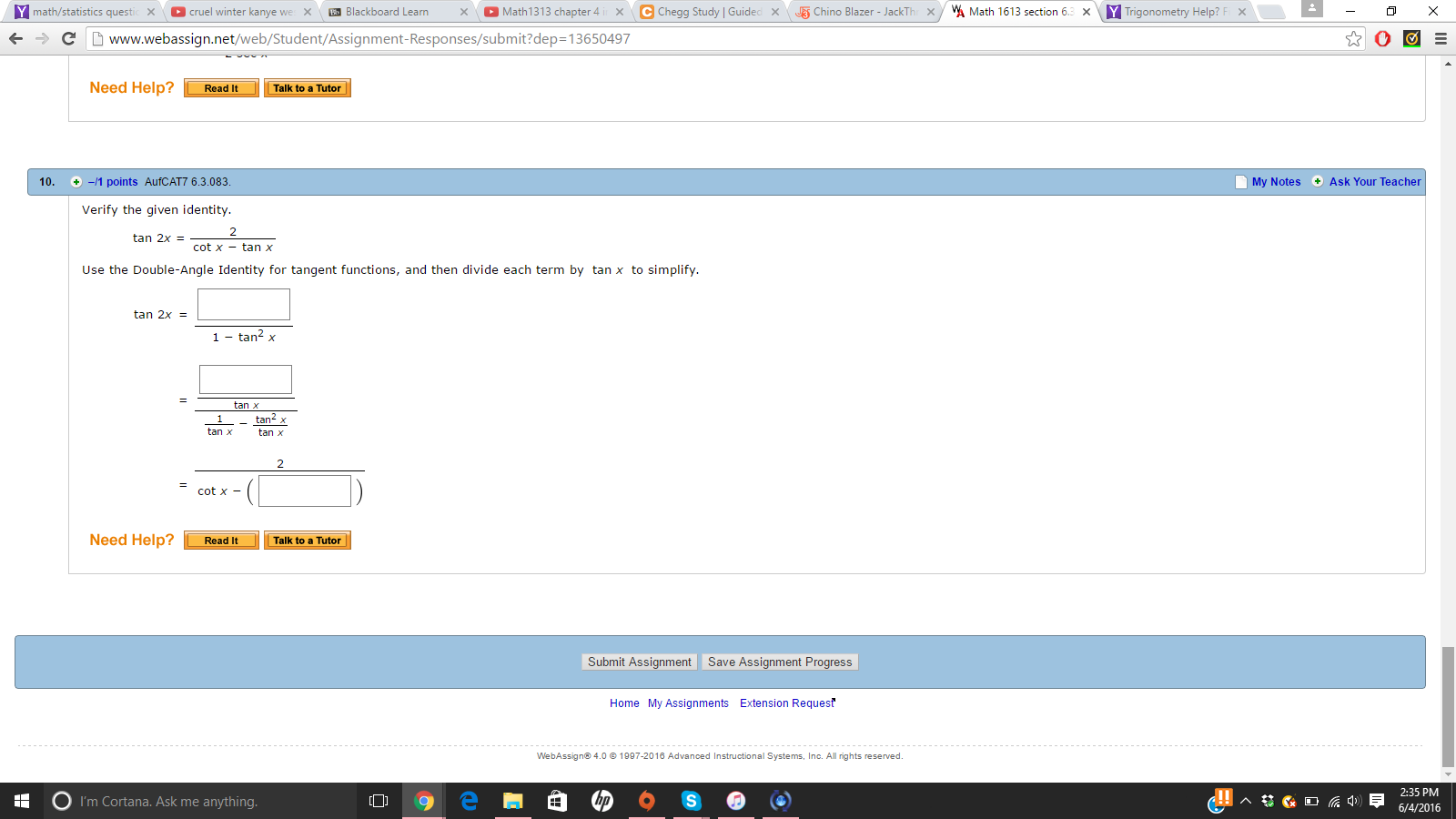



Verify The Given Identity Tan 2x 2 Cot X Tan X Chegg Com




Consider The Following Equations 1 Cosec 2x Sec 2x Cosec 2xsec 2x 2 Sec 2x Tan 2x Sec 2xtan 2x 3 Cosec 2x Tan 2x Cot 2x




1 Sin 2x Tan 2x Sec Sec 2 X Csc 2x 1 1 C Gauthmath




Moshtagh Www Konkur In




Cau D ạ Giup Minh Với Minh Cảm ơn Minh Lam Khong Rau 11 Vdc Chứng Minh 1 Cos X Cos2x A Cot X 3d Sin 2x Sinx 1 2 Sin




Trigonometry Reciprocal Identities Expii




Ppt Analytic Trig Powerpoint Presentation Free Download Id




How Do You Prove Tan 2x Secx 1 1 Secx Socratic




Tan 2x Cot 2x 2 Youtube



Solved K U 1 Differentiate The Following Choose 4 Only Marks A Y Tan 2x 1 Cot 2x B F X Xsin X 1 X C G X Sec Quot 6x Cos Course Hero




If Cos 2x Cos 4x 1 Then The Value Of Tan 4x Cot 4x Tan 2x Cot 2x Is Equal To Detailed Brainly In



Www Assignmentexpert Com Homework Answers Mathematics Answer Pdf



Cot2x To Tan2x The Student Room




E 1 Tan X C 2 Csc X 3 Csc X A 4 Cot 2x 5 Se Gauthmath




Frac D D X Left E Log Sqrt 1 Cot 2 X Right A Csc X Cot 2 X Quad B Csc X Cot X Quad C Csc 2 X Cdot Cot X D 0




How Do You Integrate Csc2x Cot2x 2 Dx Socratic



Http Www Pitt Edu Evt3 Triviums Trigonometry Trivium Pdf




1 Tan1 1 Tan2 1 Tan3 1 Tan45 2 N Brainly In Ryad Antonio Ebooks En Ligne Gratuit




Frac 11 Tan 2x Frac 11 Cot 2x 1 Gauthmath




Int 1 Tan 2x 1 Cot 2x Dx




How Do You Prove Tan 2x Secx 1 1 Secx Socratic




Prove The Identity 1 Tan 2x 1 Tan 2x 1 2sin 2x In Urdu Hindi Youtube




1 Tan 2x 1 Cot 2x 1 Tanx 1 Cotx 2 Prove That Brainly In
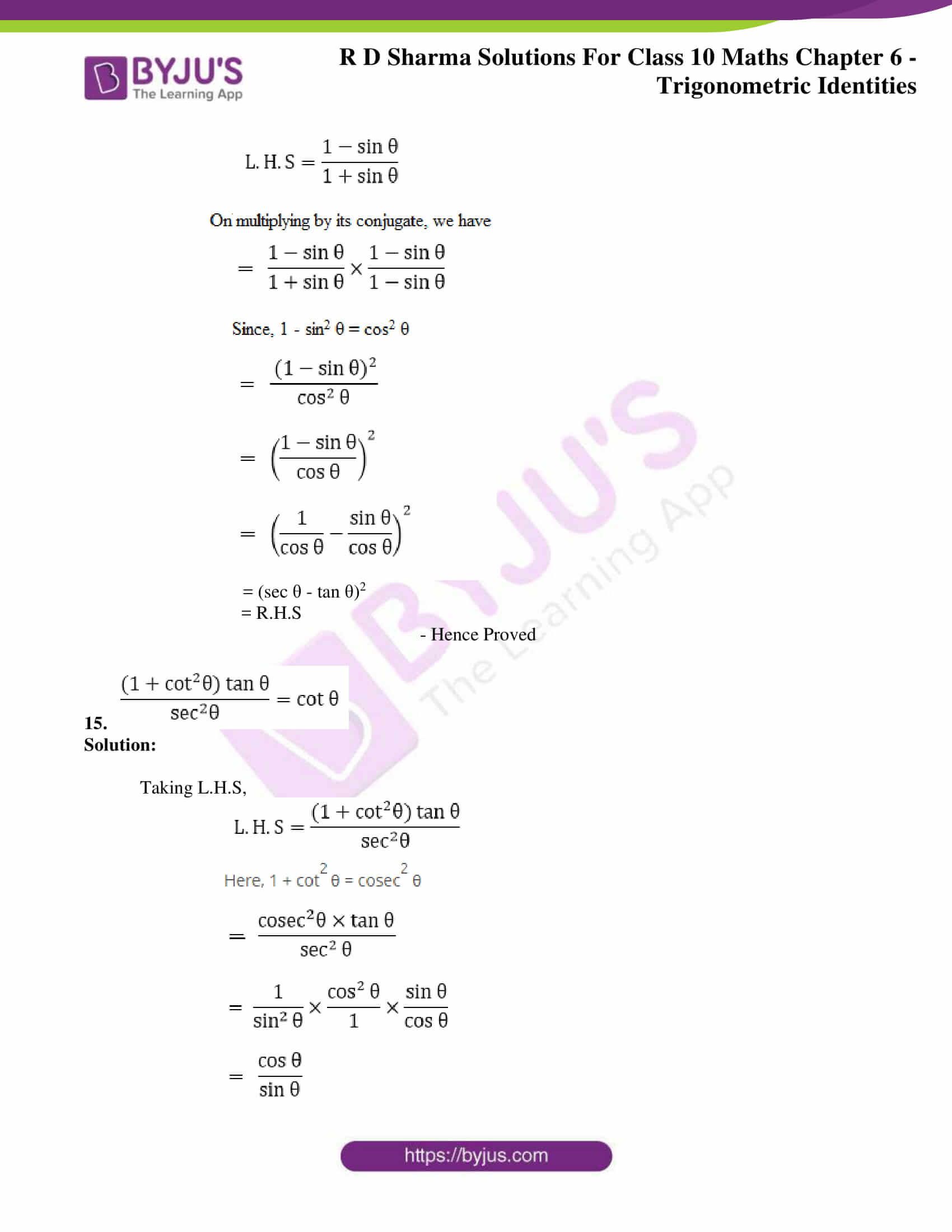



Rd Sharma Solutions For Class 10 Chapter 6 Trigonometric Identities Exercise 6 1 Get Pdf




1 Cot 2x S A Sin 2x B Cos 2x C Csc 2x D Sec Gauthmath



Search Q Trig Identities Tbm Isch



Pmt Physicsandmathstutor Com Download Maths A Level C3 Topic Qs Edexcel Set 1 C3 trigonometry trigonometric identities Pdf




Evaluate Int Cosec 3 2x 1 Cot 2x 1 Dx




5 Tan X Tan 2x Dx Select One A 1 2 H Tan 2 X Chegg Com




Int Cos8x 1 Tan2x Cot2x Dx Youtube



Http Theprofessorshut Weebly Com Uploads 2 3 2 7 Pc Chap 6 Sect 2 Sum Difference And Cofunction Identities Pdf
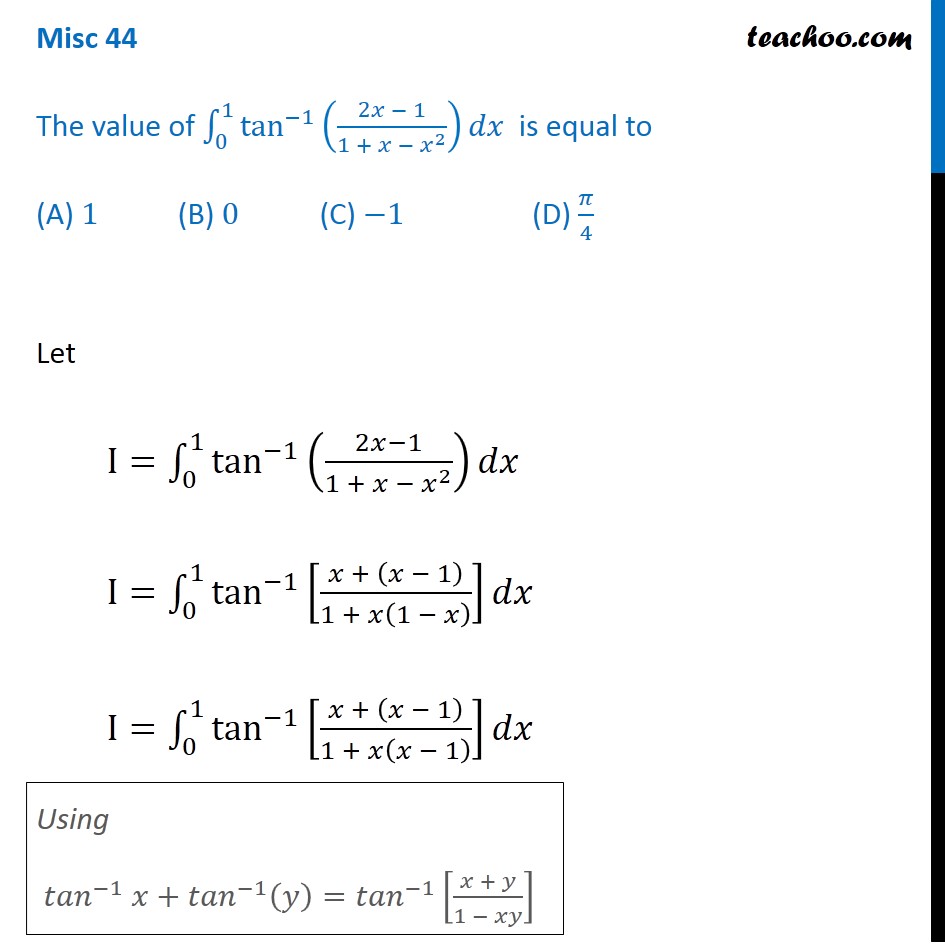



Misc 44 Value Fo Tan 1 2x 1 1 X X2 Dx Is Miscellaneous




Integration Trig Identities Ppt Download



Trigonometry Identity 1 Cot 2 X Csc 2 X Youtube




Sin X 2 Tan X 2 1 Tan 2 X 2 Eraoco



0 件のコメント:
コメントを投稿